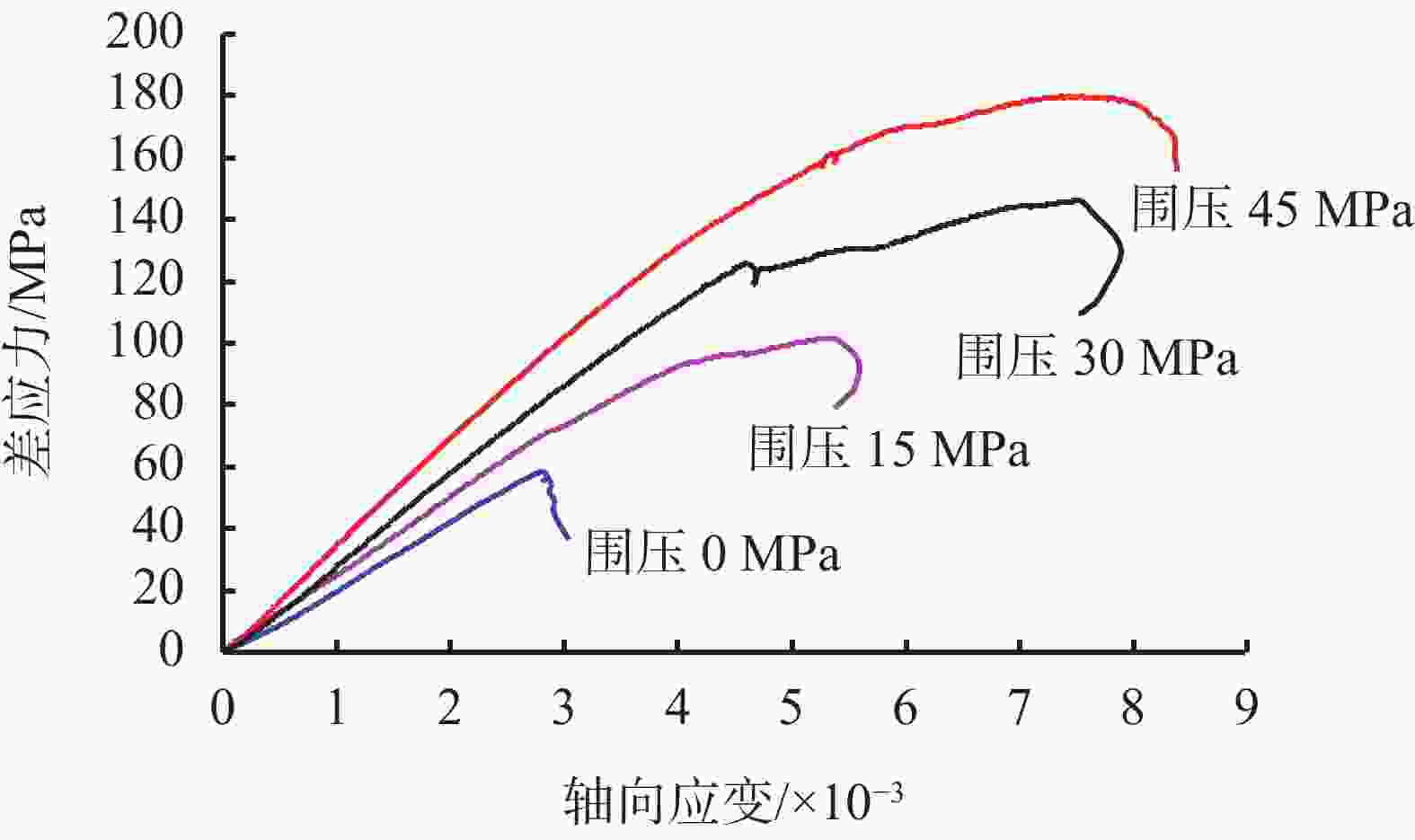
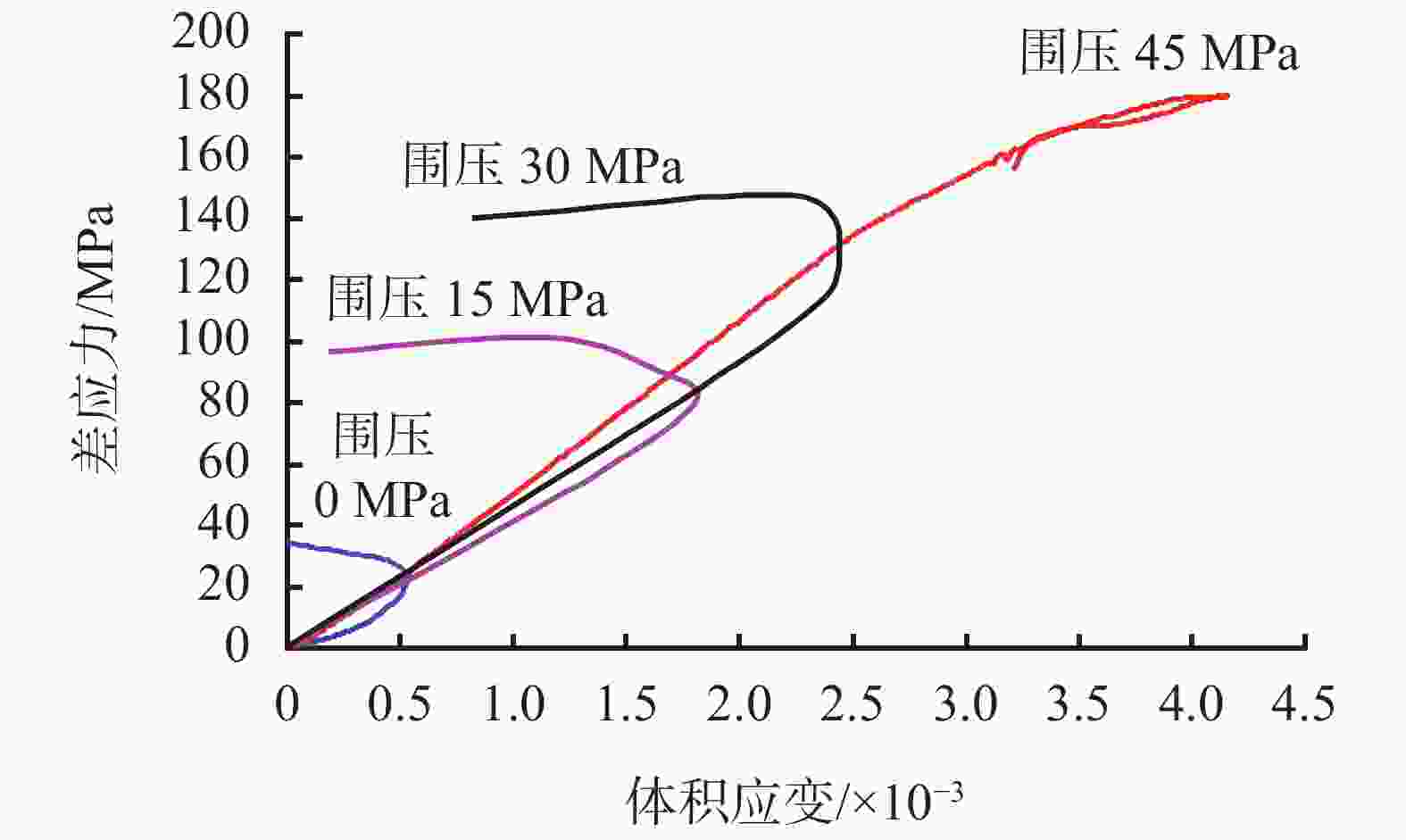
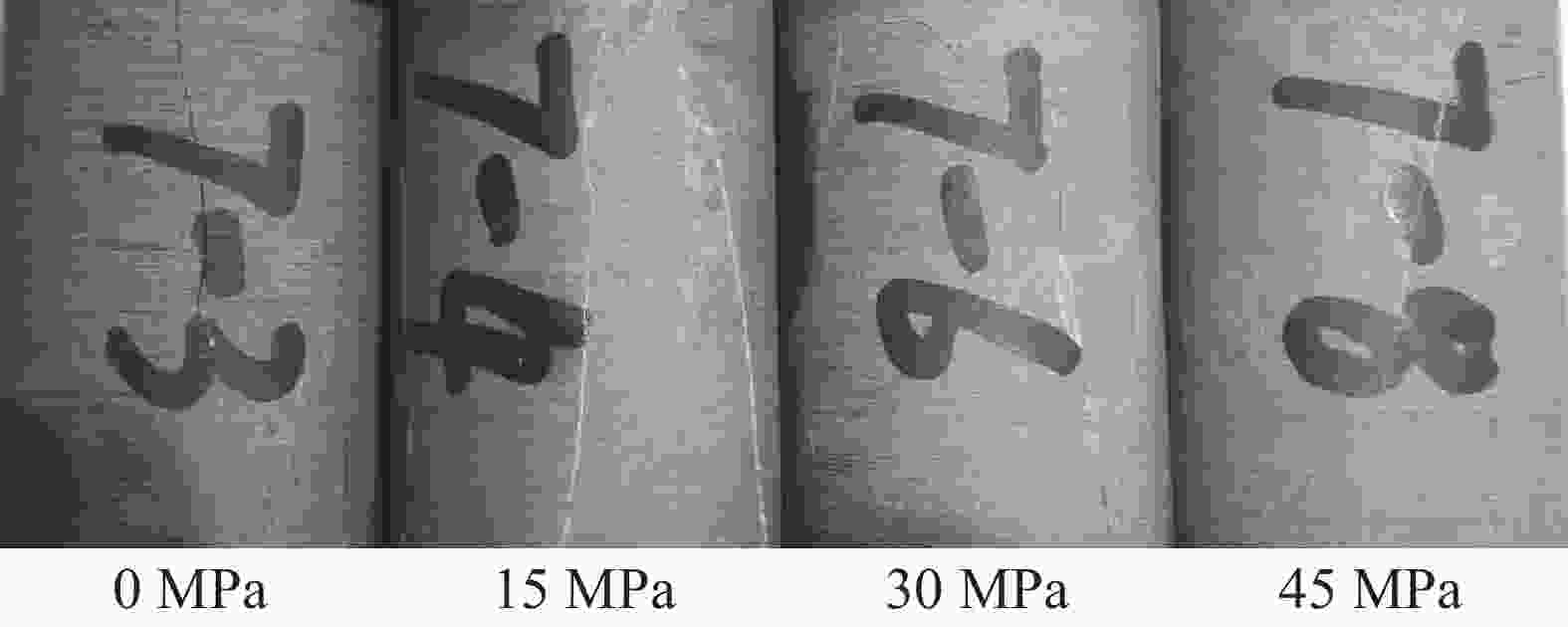
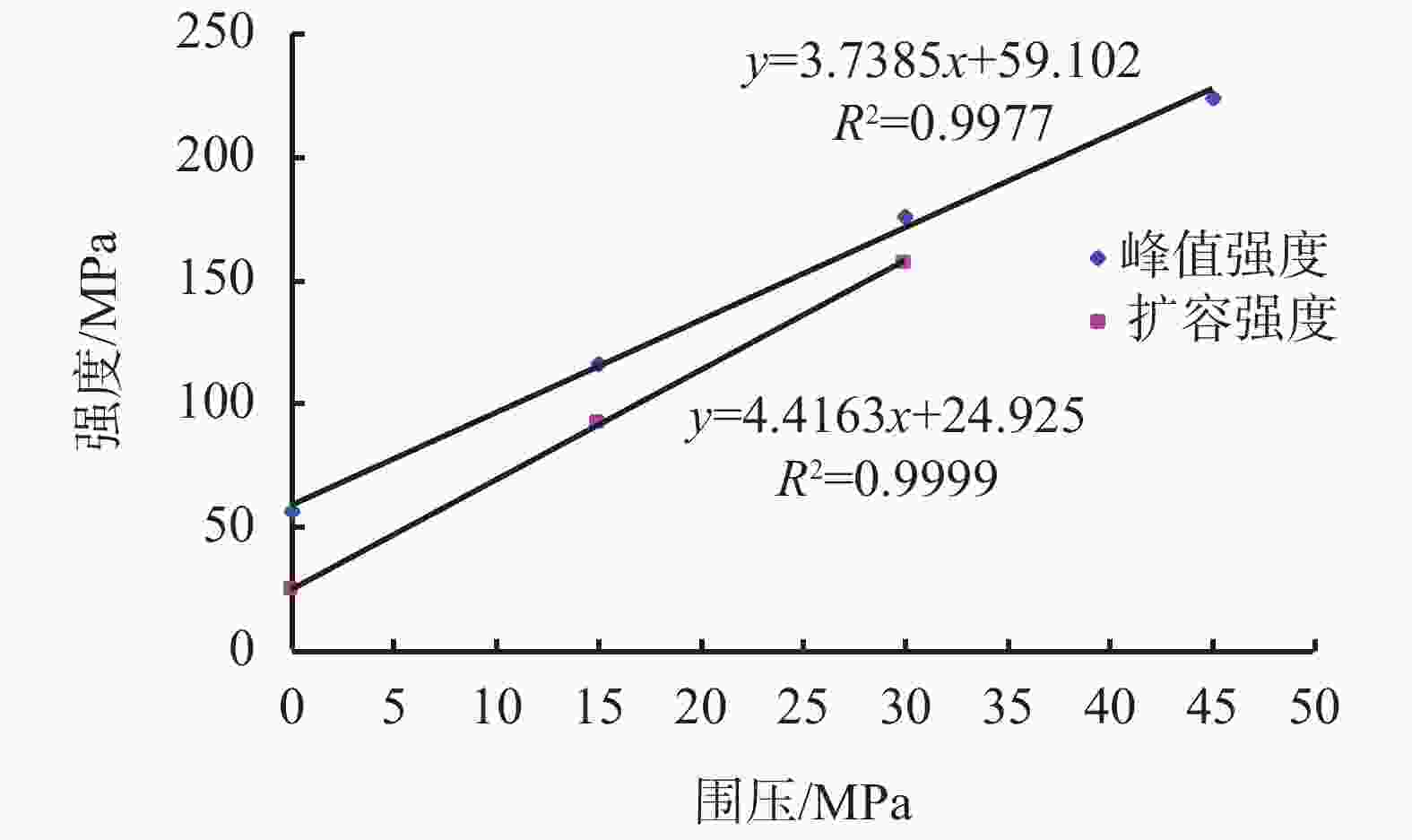
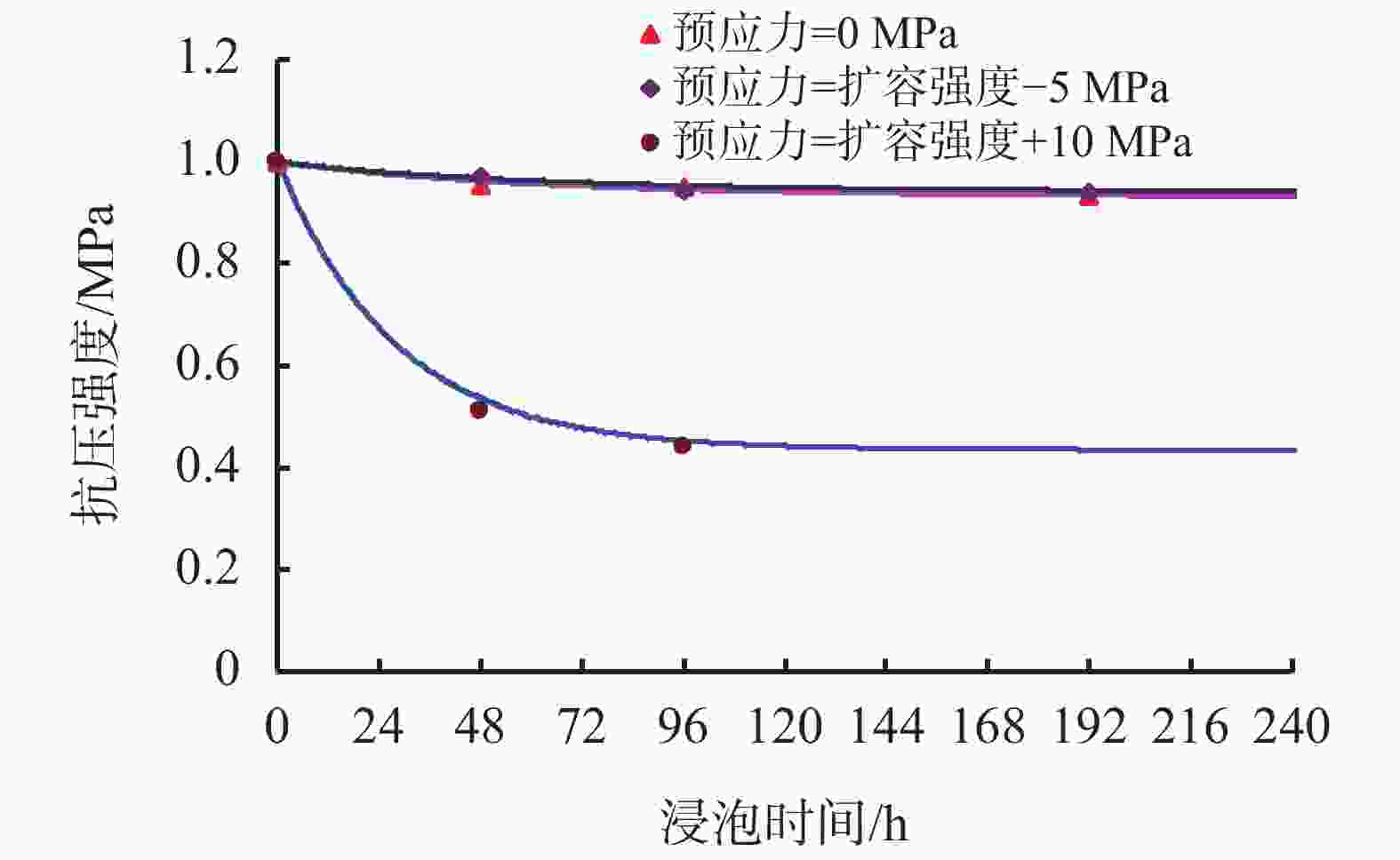
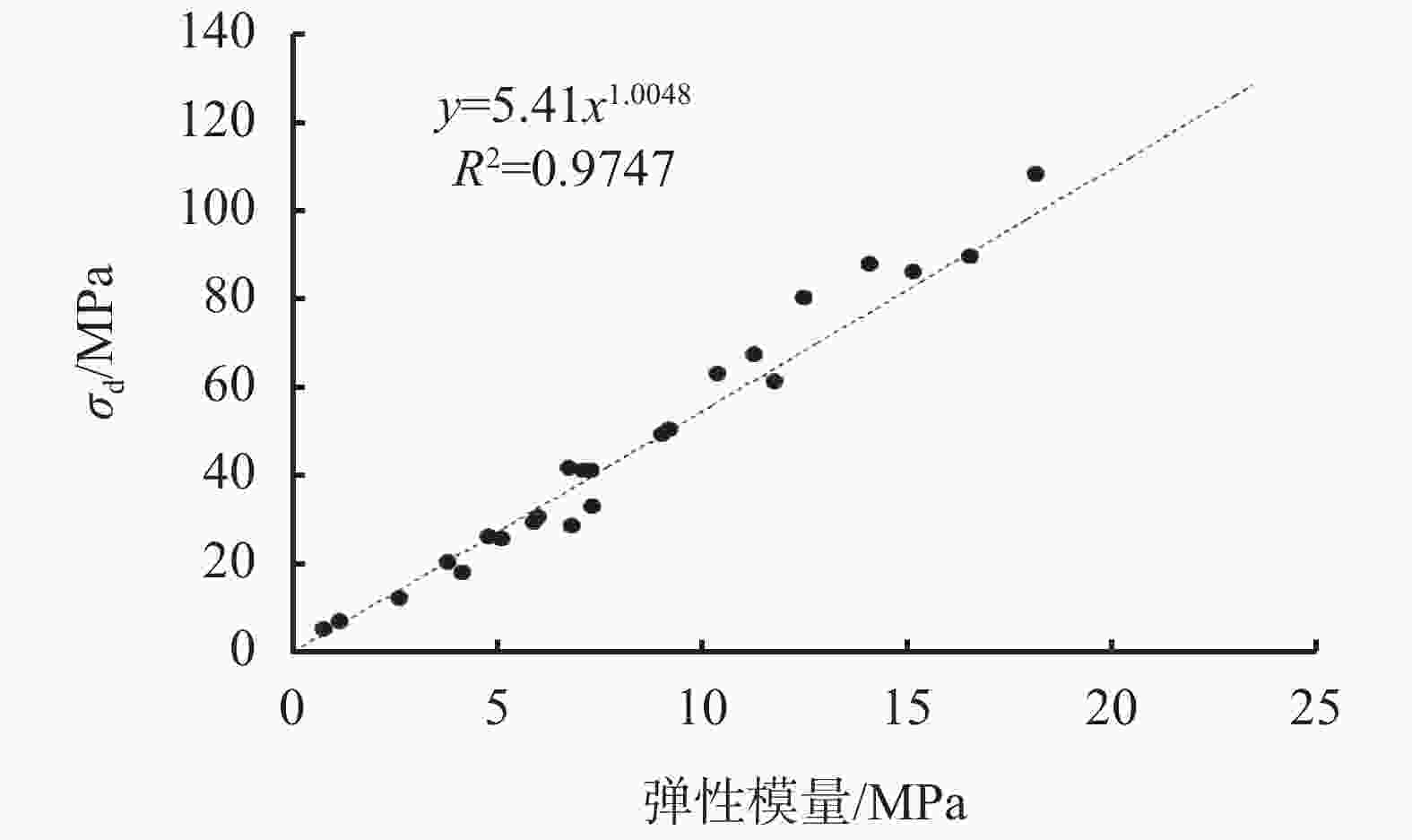
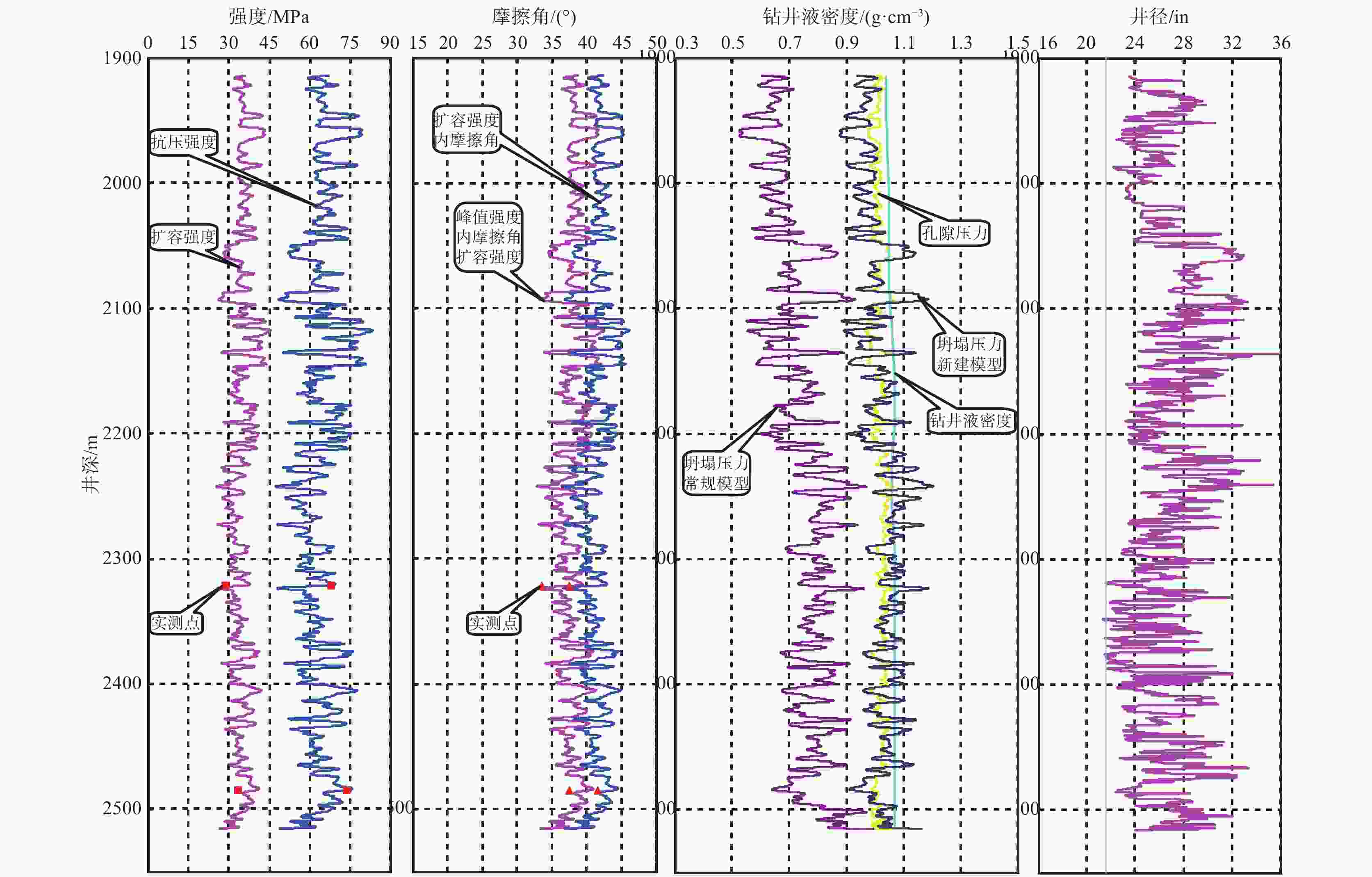
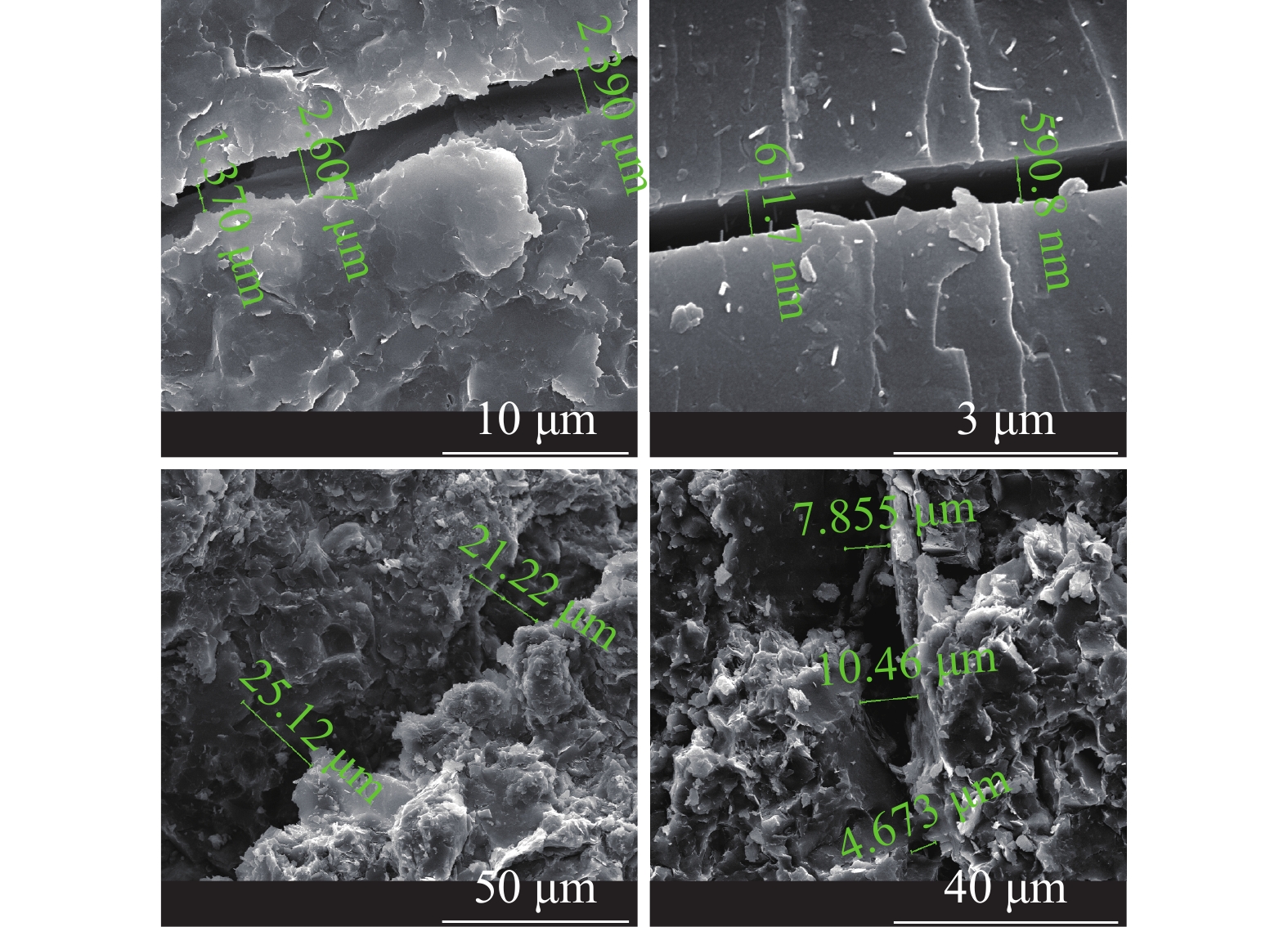
Abstract: Under low confining pressures, a hard brittle mudstone is easy to dilate before the stress acted on the mudstone reaches its peak value. When the stress is greater than the dilation strength of the shale, the weakening of the mudstone by the hydration of the drilling fluid becomes fast and outstanding, resulting in borehole collapse. To avoid borehole collapse, a reasonable mud weight should be used. Through laboratory experiment and theoretical analysis, studies were conducted on the factors such as the fabric characteristics, hydrophysical properties, deforming patterns and strength criteria of hard brittle mudstones, as well as the change of strengths of mudstones after unloading prestress in a drilling fluid. A computation model and parameter computing method based on rock dilation strength criteria were developed for the collapse pressure of a borehole penetrating hard brittle mudstones, and a case study was conducted. The study results show that the mud weight is greater than the collapse pressure calculated on the basis of peak strength criteria and is lower than the collapse pressure calculated on the basis of dilation strength criteria, the formation around the borehole is in a state of dilation, and stress-induced micro fractures are generated around the wellbore, which stimulates drilling fluid hydration, and this is the root cause for borehole collapse. Based on this knowledge, mud weight determined on the basis of dilation strength criteria is more reasonable.