Simulation Study on Movement of Solidifying LCMs for Controlling Mud Losses into Fracturing Formations
-
摘要: 固化类堵漏材料常用于裂缝性地层恶性漏失堵漏,在裂缝近井壁处形成完整的固结段塞,是堵漏成功的前提。固化类材料进入井筒后难免会与地层流体发生共混,堵漏浆-地层流体两相体积分布随着空间和时间变化,与流体理化性质、施工参数、裂缝几何形貌等有密切关系。为此,采用CFD仿真模拟方法,研究了固化堵漏浆密度和流变参数对堵漏浆裂缝体积分布及流速的影响规律,几何模型选择三维井筒-垂直裂缝模型,模拟压差为1.9 MPa,堵漏浆入口流速为2.5 m/s,两相流模型为VOF模型,井筒和裂缝中原始流体为水基钻井液。模拟结果表明,堵漏浆密度和动切力对其在井筒和裂缝中运移影响少,稠度系数和流性指数对堵漏浆裂缝驻留性能影响显著。稠度系数或流性指数越高,堵漏浆裂缝中流速越小,体积分数越高,低于临界值后,裂缝中将一直以堵漏浆-钻井液共混流体存在。流性指数相比稠度系数对于堵漏浆裂缝驻留能力影响更为显著,牛顿流体和剪切增稠型堵漏浆更利于在裂缝中形成完整段塞。该仿真模拟工作为固化堵漏浆流变性优化提供一定理论基础,有利于提升固化堵漏技术一次成功率。Abstract: Solidifying lost circulation materials (LCMs) are always used to control severe mud losses into fractured formations, they control mud losses by forming whole plugs near the borehole wall in the fractures. The solidifying LCMs entering the wellbore often inevitably are mixed with formation fluids. The volume distribution of the LCM slurry and the formation fluids changes with time and location, and is closely related to the physical-chemical properties of the formation fluids, the operation parameters and the geometry of the fractures. Using the computational fluid dynamics (CFD) method, the effects of the density and rheology of solidifying LCM slurries on the volume distribution of the LCM slurry and the formation fluids in the fractures and the flow velocity of the fluids were studied. In this study a 3D wellbore-vertical fracture model was used, the pressure differential used in the simulation was 1.9 MPa, the flow rate of the LCM slurry at the entry of the fractures was 2.5 m/s, the two-phase model was a VOF model, and the in-situ fluids in the wellbore and the fractures were a water based drilling fluid. The simulation results showed that the density and the yield point of the LCM slurry have little effect on the movement of the LCM slurry in the wellbore and the fractures, while the consistency index and the flow index significantly affected the residency of the LCM slurry in the fractures; the higher the consistency index and/or flow index, the lower the flow rate of the LCM slurry inside the fractures, and the higher the volume fraction of the LCM slurry inside the fractures. When the flow rate of the LCM slurry is less than the critical flow rate, the fractures will always be filled with the mixture of the LCM slurry and the drilling fluid. Compared with consistency index, flow index plays a more important role in affecting the residency of LCM slurries in formation fractures. LCM slurries of the Newtonian fluids and the shear-thickening fluids more easily form whole plugs in a fracture. This CFD simulation provides a theoretical basis for the optimization of the rheology of solidifying LCM slurries and is beneficial to the success rate at first try of controlling mud losses with the solidifying LCM slurries.
-
0. 引言
裂缝性地层恶性漏失是油气钻井重大工程技术难题之一,严重影响勘探开发进程[1]。水泥、交联聚合物、树脂等固化堵漏材料常用于裂缝性地层恶性井漏堵漏,相比于桥堵颗粒,此类材料具有对裂缝通道适应性好、固化后承压效果好的优点。固化材料若要发挥堵漏作用,“停得住”、“填得满”是首要条件,现场堵漏应用中常出现无法形成段塞的情况,需要反复施工[2-3]。
出钻杆的固化堵漏浆将与井筒和裂缝中流体发生两相甚至三相共混,并向裂缝深部运移,固化堵漏浆在裂缝中的驻留、填充情况,将直接影响能否固化和固化后的封堵效果。显然,堵漏浆-地层流体在井下的多相流过程与流体理化性能、施工参数、裂缝通道尺寸等有关。当前,固化类材料的机理研究主要集中在固化时间[4]和强度[5]的调控,揭示固化剂、交联剂、缓凝剂分子结构对固化时长的影响规律[6-8],针对挤注过程中的井下运移机理研究基本空白,堵漏浆理化性能和施工参数优化缺乏科学指导。
建立三维井筒-裂缝几何模型,采用VOF两相流计算模型[9],定量分析了堵漏浆密度和流变性对堵漏浆在裂缝中的流速和体积分数的影响规律,为固化堵漏浆的性能和施工参数优化提供理论支持。
1. 仿真模型
1.1 几何模型
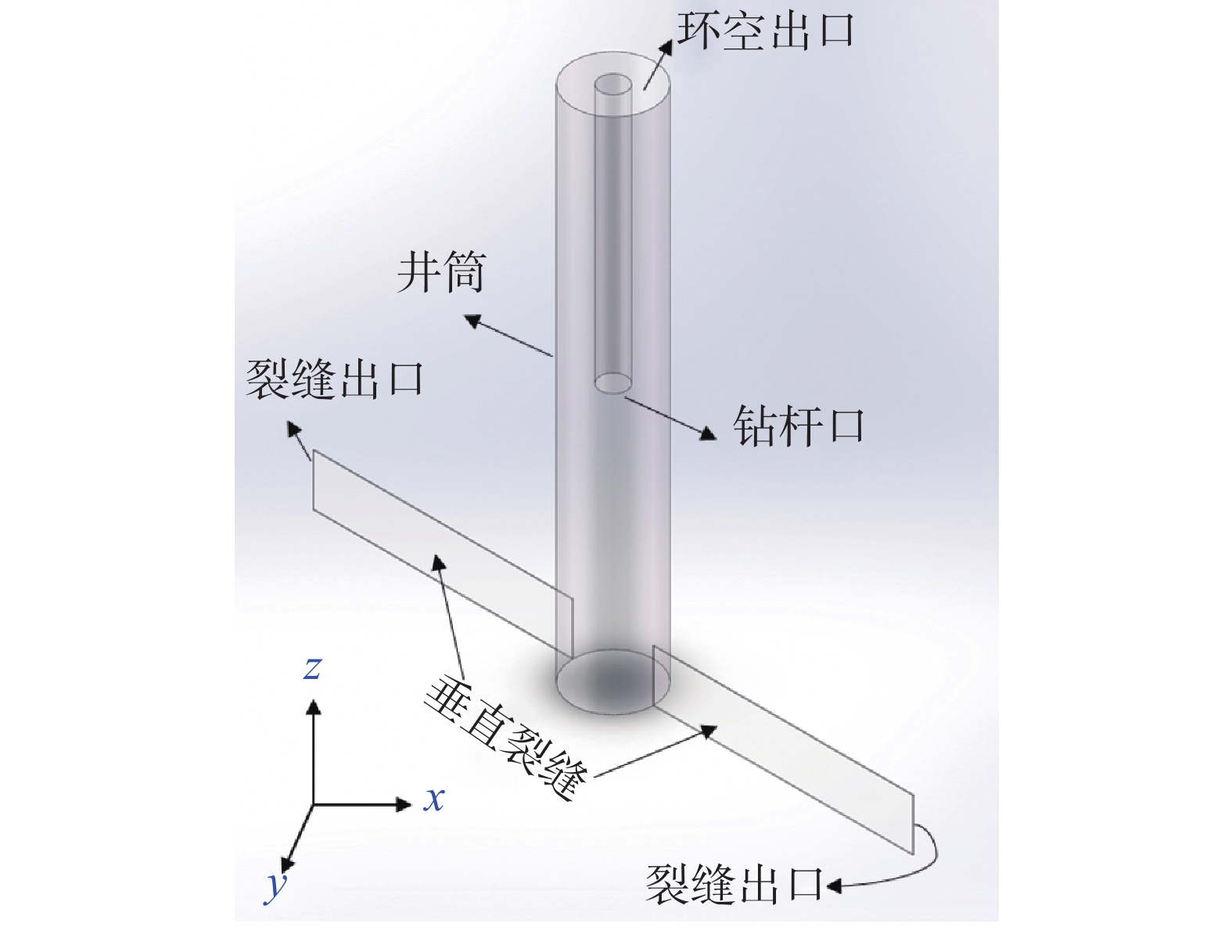
仿真模型为三维井筒,井筒底部联通两条对称的垂直裂缝,顶部带有钻杆,如图1所示。整个模型沿裂缝横截面(x-z平面)对称,井筒和钻杆直径参考钻井实际尺寸,分别为311 mm和100 mm;井筒长度设置为3 m,钻杆长度为1 m;裂缝高度20 cm,宽度3 mm,长度1 m;网格为四面体网格。
1.2 边界条件
整个仿真模型设置1个钻杆入口,1个环空出口和2个裂缝出口。入口设置为速度入口边界,固定为2.5 m/s;出口为压力边界,裂缝出口压力固定为0.1 MPa;入口和环空出口压力设为相等,均为2 MPa。井筒和裂缝中流体为钻井液,入口流体为堵漏浆,2种流体均为赫-巴流型。水基钻井液密度为1.2 g/cm3,动切力τ0为4 Pa,稠度系数K为0.65 Pa·sn,流性指数n为0.89。
堵漏浆流变性、密度参数设置单因素考察表,见表1,逐一分析不同参数对堵漏浆井下运移状态的影响。
表 1 三维井筒-裂缝仿真模型参数 梯度设置 堵漏浆密度/(g·cm−3) 1.1、1.2、1.3、1.4、1.5 堵漏浆流变性能 τ0/Pa 0、1.5、3.0、5.0、6.5 K/Pa·sn 0.5、2.0、3.5、5.0、6.5 n 0.4、0.5、0.6、0.7、1.0、1.3 1.3 求解方法
参考固井水泥浆环空顶替效率仿真模拟计算方法[10-13],整个计算过程为瞬态计算,选择VOF两相流模型,模拟步长0.1 s,模拟时长30 s。其基本方程如下,假设有不可压缩两相流体堵漏浆和钻井液,其体积分数、体积平均密度和黏度满足公式(1)~(3)。连续性方程和动量控制方程分别为公式(4)和(5)。其中,
$ {\alpha _{\rm{l\,cm}}} $ 和$ {\alpha _{\rm{wbd}}} $ 分别为堵漏浆及钻井液体积分数;$\rho_{\rm{l\,cm}} $ 、$ {\rho _{\rm{wbd}}} $ 和$ \rho $ 分别为堵漏浆、钻井液及体积平均密度,kg/m3;$ {\mu _{\rm{l\,cm}}} $ 、$ {\mu _{\rm{wbd}}} $ 和$ \mu $ 分别为堵漏浆、钻井液及体积平均黏度,Pa·s;u为速度矢量,m/s;p为压强,Pa;f为体积力,N/cm3。$$ \alpha_{\rm{l\,cm}}+\alpha_{\text {wbd }}=1 $$ (1) $$ \alpha_{\rm{l\,cm}} \rho_{\text {l\,cm }}+\alpha_{\text {wbd }} \rho_{\text {wbd }}=\rho $$ (2) $$ \alpha_{\rm{l\,cm}} \mu_{\text {l\,cm }}+\alpha_{\text {wbd }} \mu_{\text {wbd }}=\mu $$ (3) $$ \frac{{\partial \rho }}{{\partial t}} + \frac{\partial }{{\partial {x_i}}}(\rho {u_i}) = 0 $$ (4) $$ \rho \left(\frac{\partial u}{\partial t}+(u{\cdot}\nabla )u\right)=-\nabla p+\rho f+\mu {\nabla }^{2}u $$ (5) 2. 结果分析
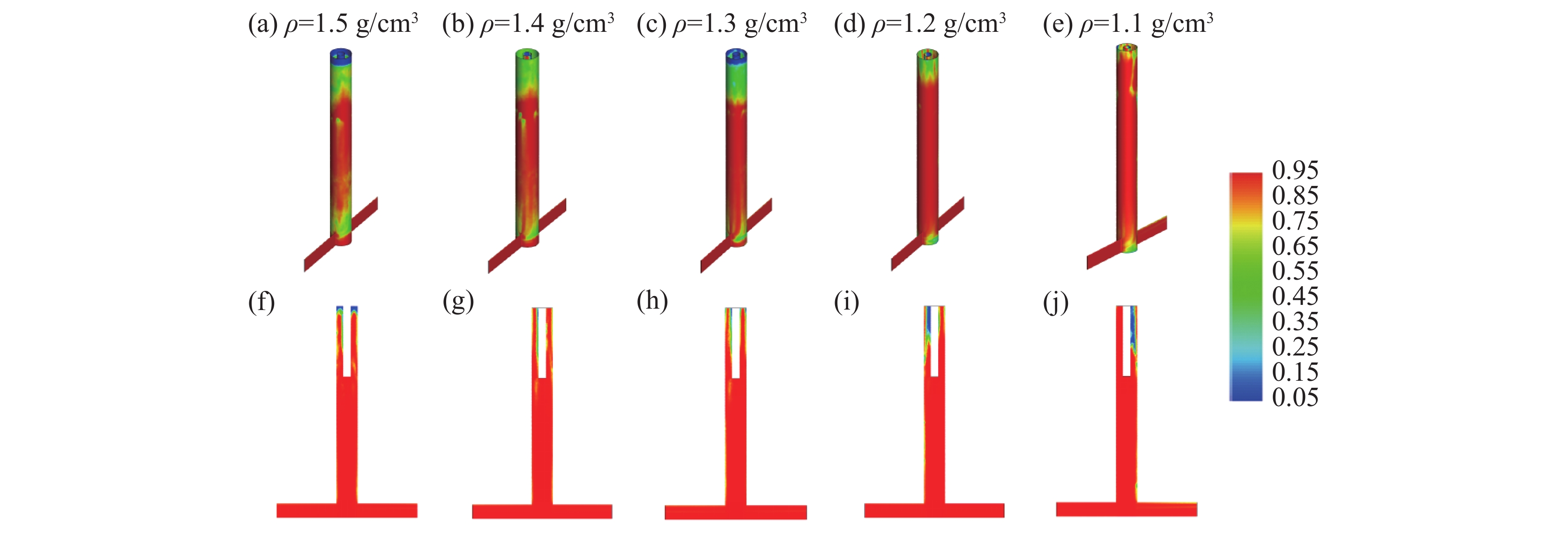
2.1 堵漏浆密度影响
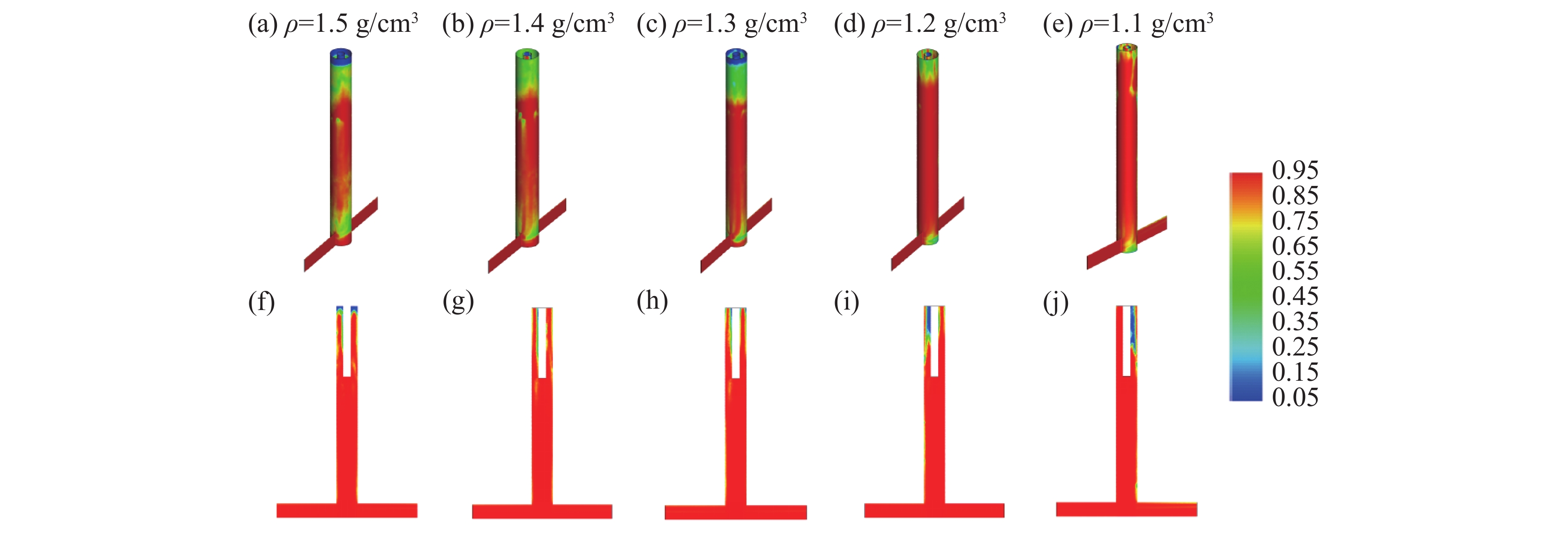
考察密度对堵漏浆井下运移影响时,堵漏浆动切力τ0为5 Pa,稠度系数K为6.5 Pa·sn,流性指数n为0.7。
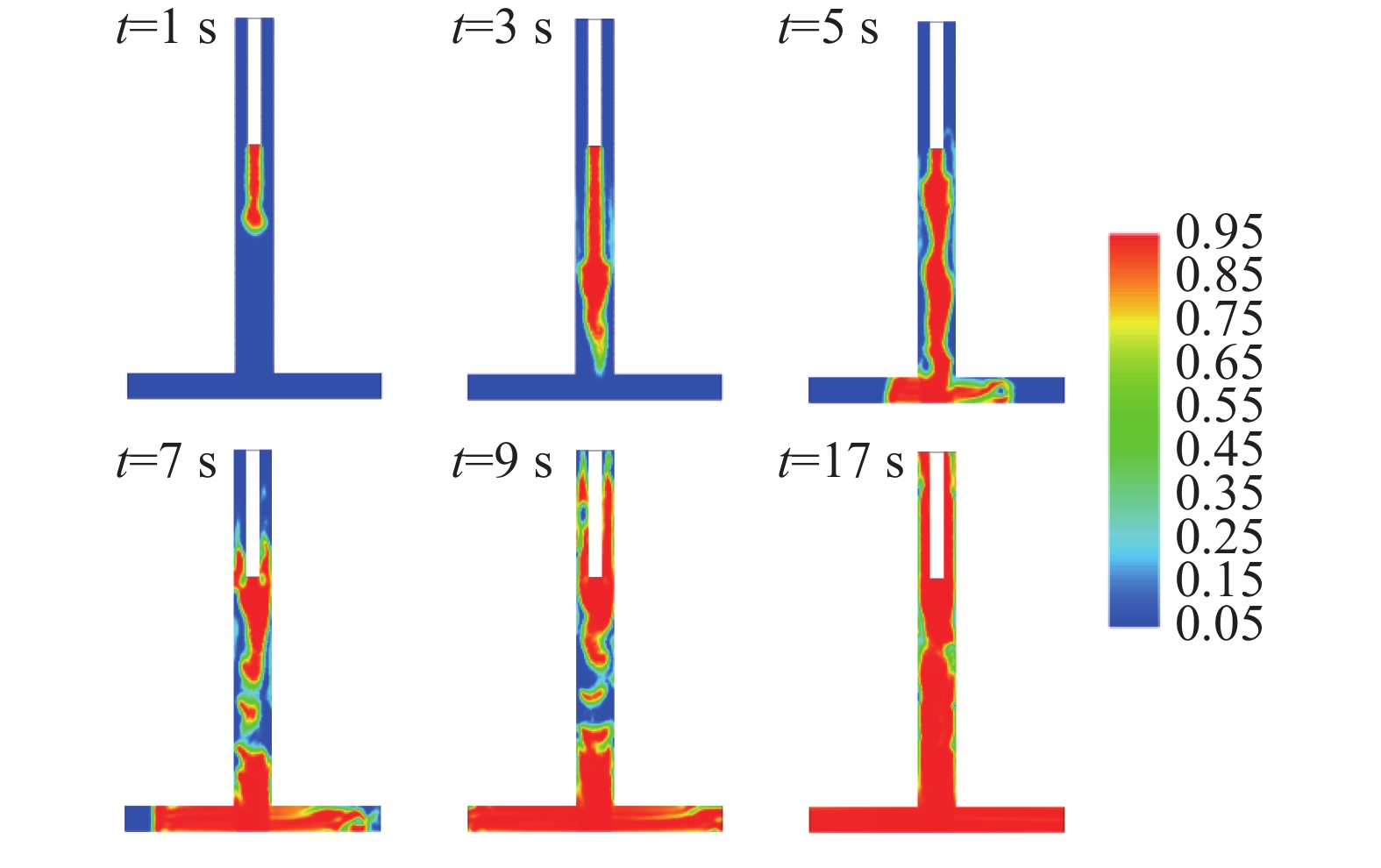
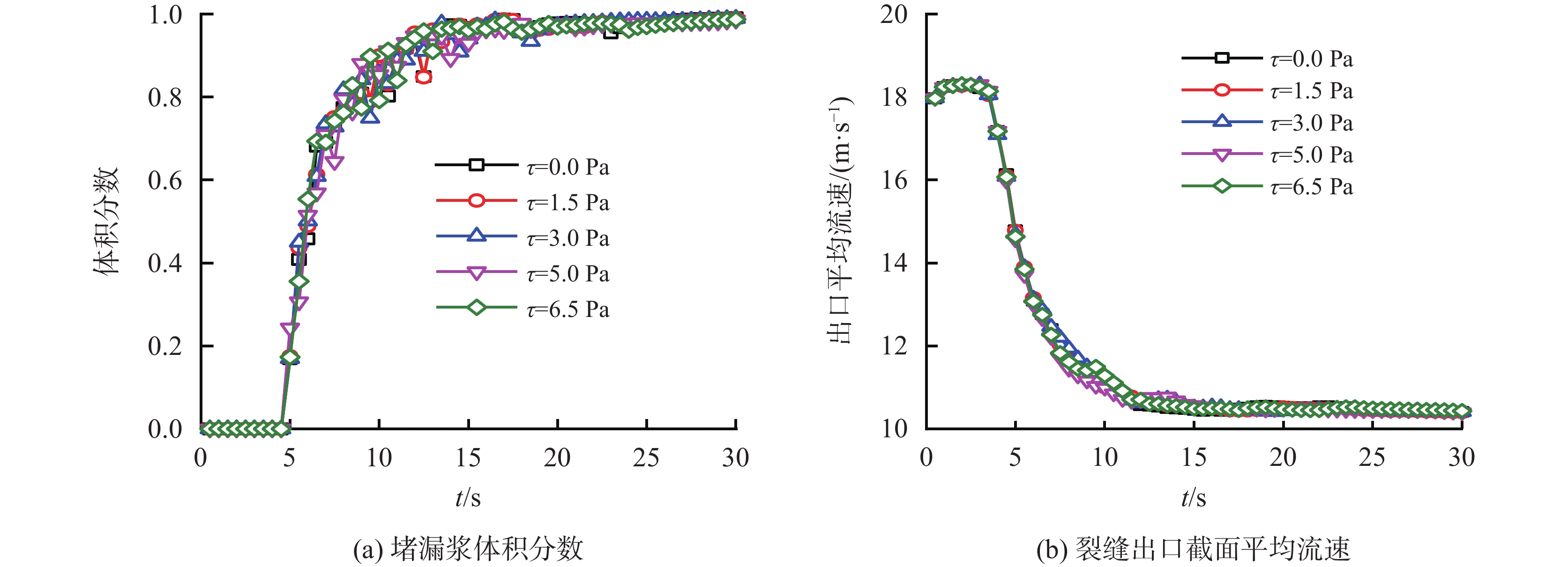
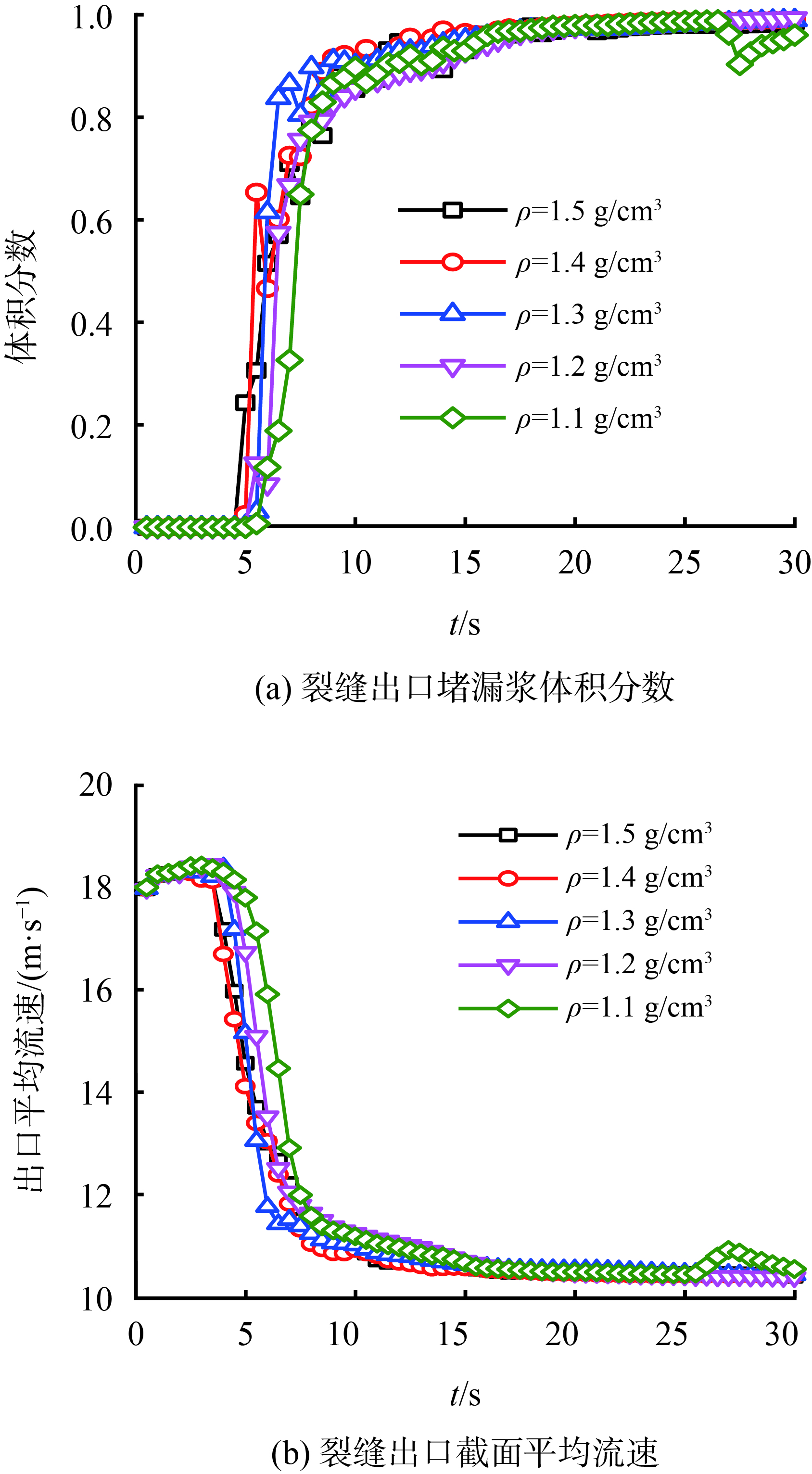
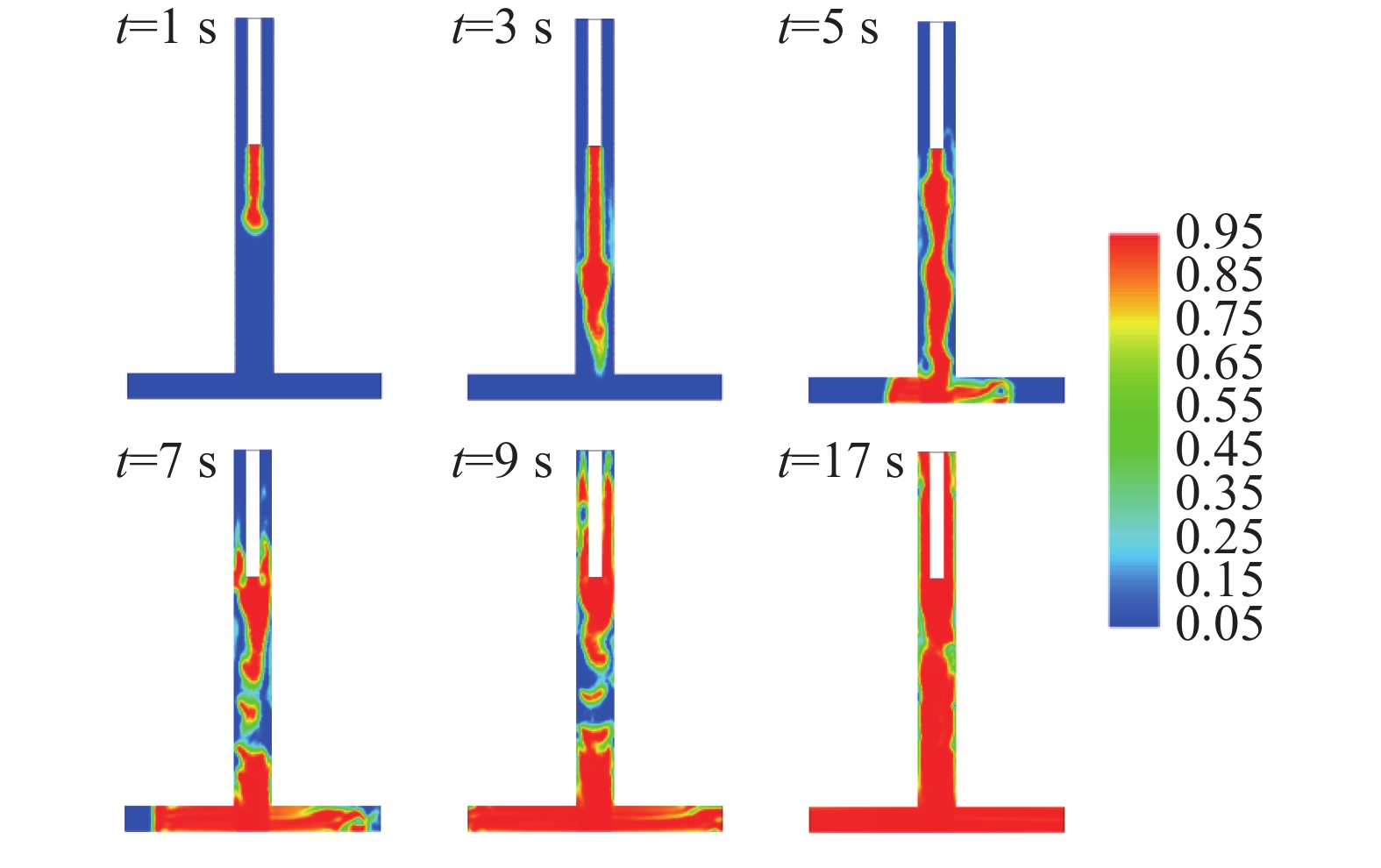
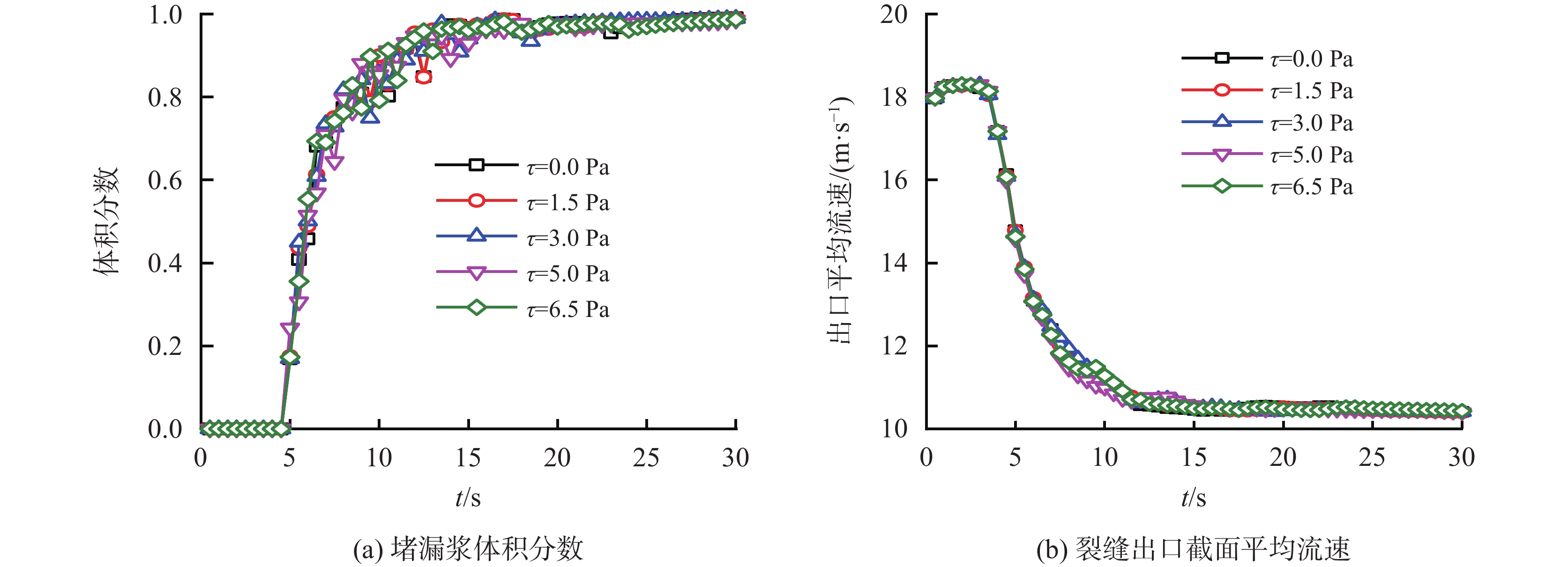
不同密度堵漏浆裂缝驻留能力基本一致。如图2(a)所示,不同密度堵漏浆在裂缝出口处体积分数随时间变化趋势基本一致,5 s后裂缝出口堵漏浆体积快速上升,10 s后裂缝出口堵漏浆体积稳定在90%以上,30 s后基本达到100%,说明10 s后裂缝中基本为纯堵漏浆流体流动。如图2(b)所示,裂缝出口端平均流速先增高,此时流出液体主要为低黏度钻井液;5 s后堵漏浆混合段达到裂缝出口端,黏度增加,平均流速快速下降;10 s后为出口端基本为堵漏浆,裂缝出口平均流速基本稳定在10 m/s,堵漏浆密度对裂缝出口流速基本没有影响。图3展示了t=30 s时井筒和裂缝中两相流体状态,密度为1.1~1.5 g/cm3堵漏浆均能填裂缝和钻杆下部井筒。图4展示了不同时刻井筒和裂缝中两相流状态,堵漏浆-钻井液流体共混段呈现“裂缝-井筒底部-环空”的移动趋势。
2.2 稠度系数影响
考察稠度系数影响时,动切力τ0和流性指数n分别固定为5 Pa和0.7。
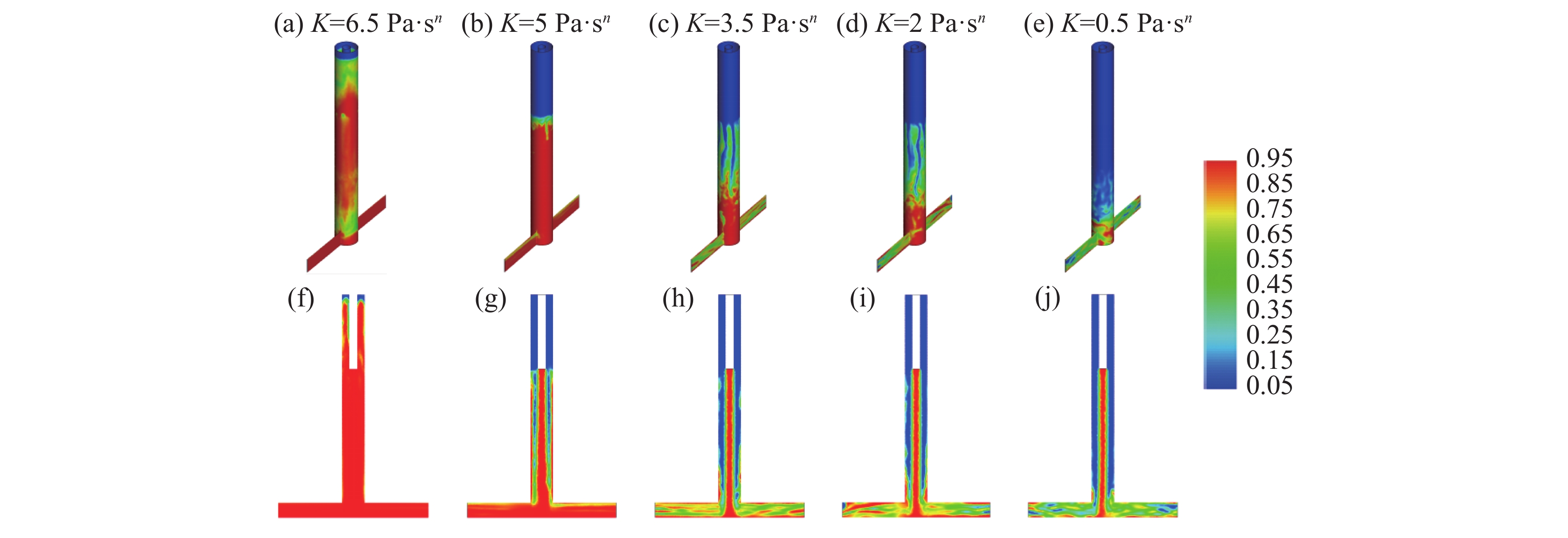
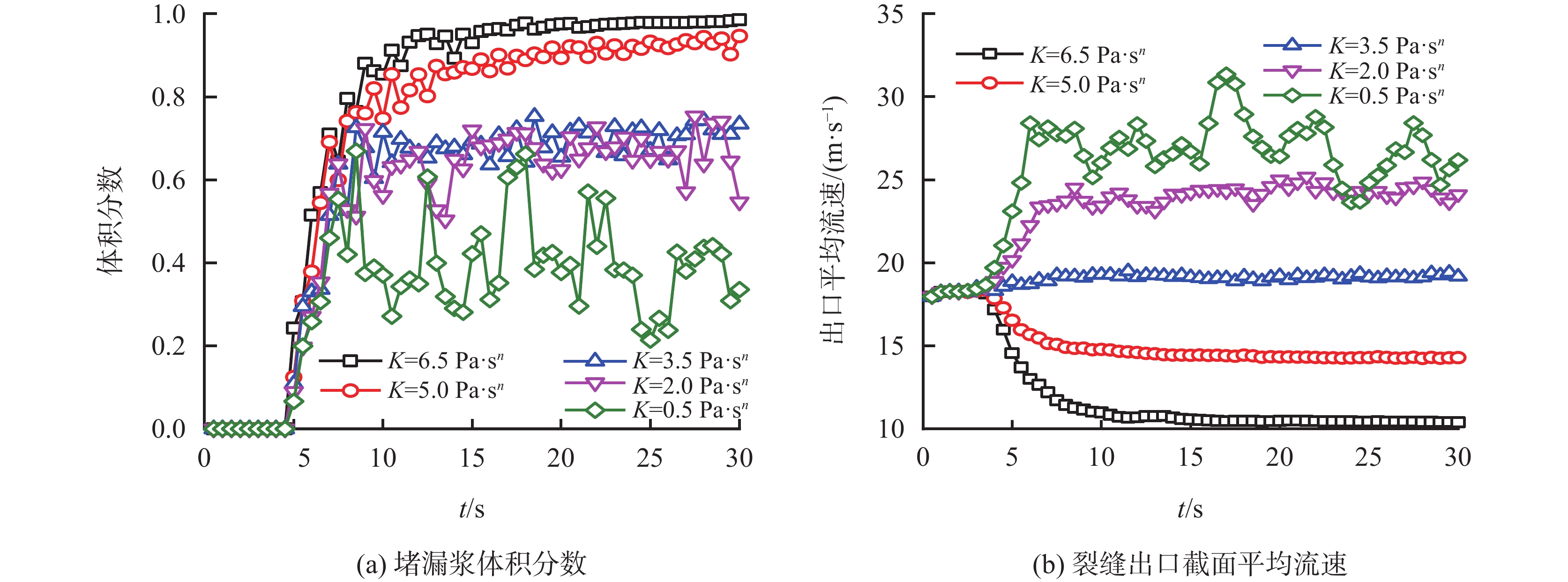
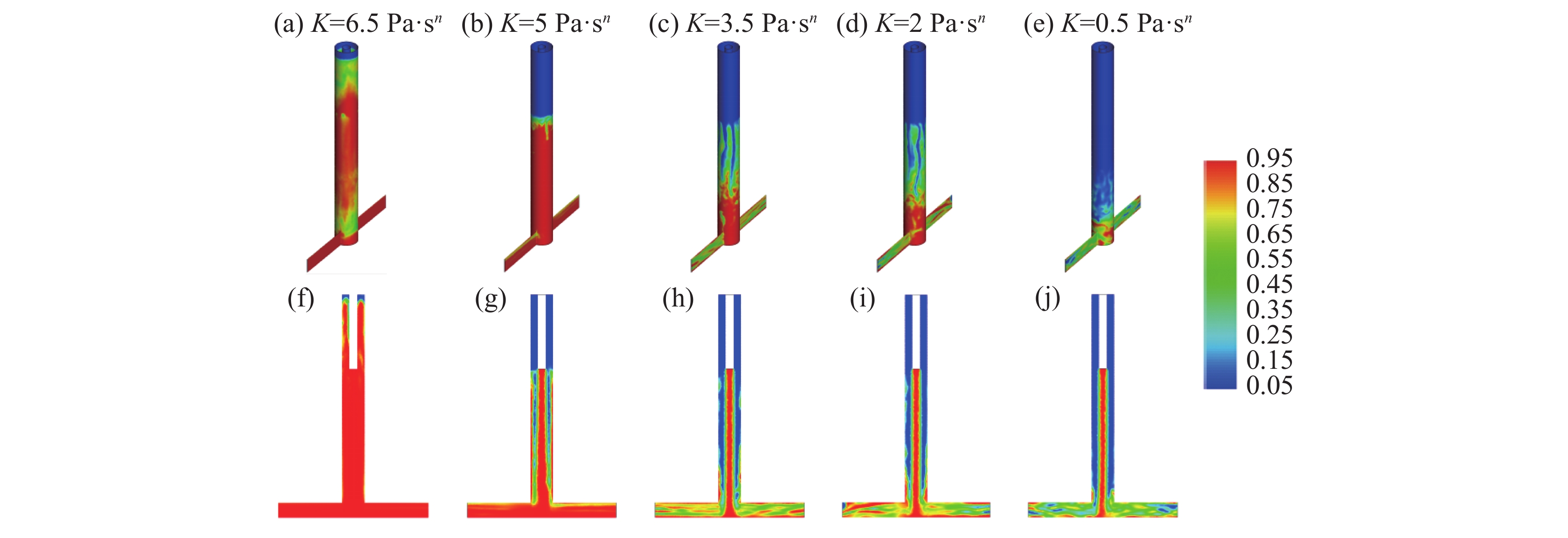
稠度系数对堵漏浆裂缝中驻留能力影响显著,稠度系数K越小,裂缝出口流速越大,堵漏浆驻留能力越差,甚至无法在裂缝中形成段塞。如图5所示,t=30 s时,K为6.5和5 Pa·sn时,堵漏浆可以在裂缝中形成完整的段塞,但K=5 Pa·sn的堵漏浆在钻杆下部井筒无法形成完整的段塞;K低于5 Pa·sn后,裂缝和井筒中全部为共混的堵漏浆,无法完整填充漏失通道和井筒。
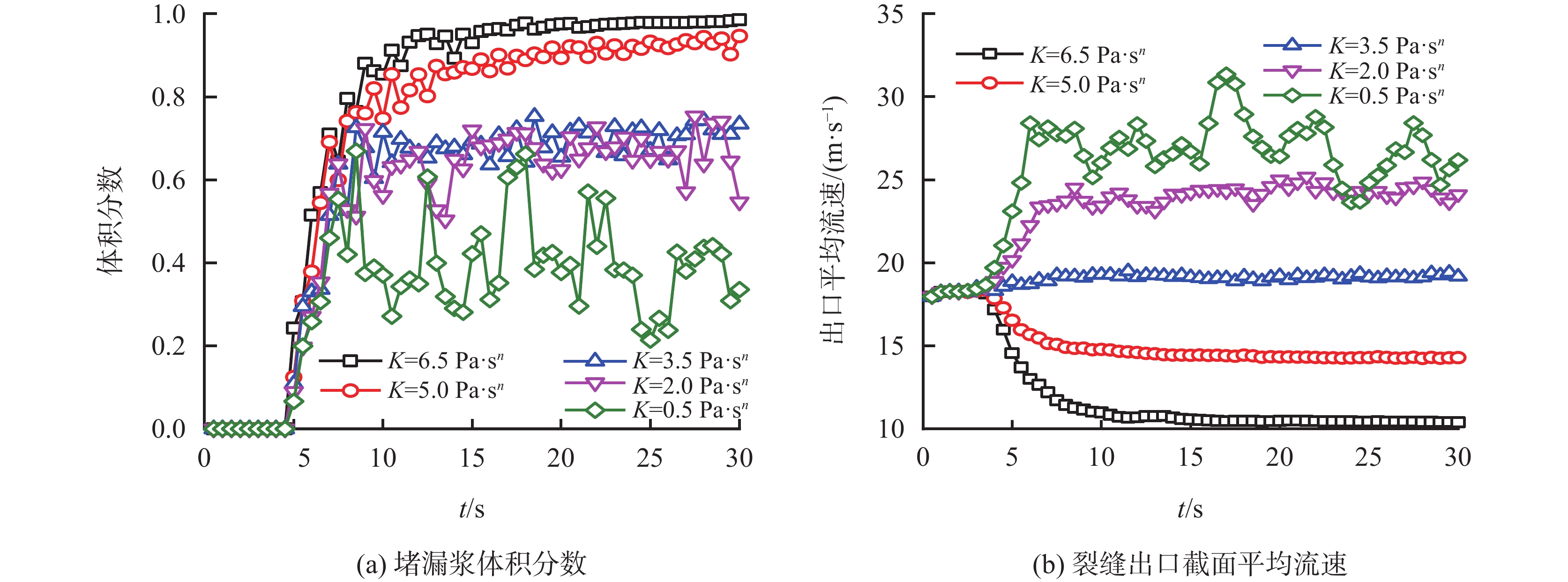
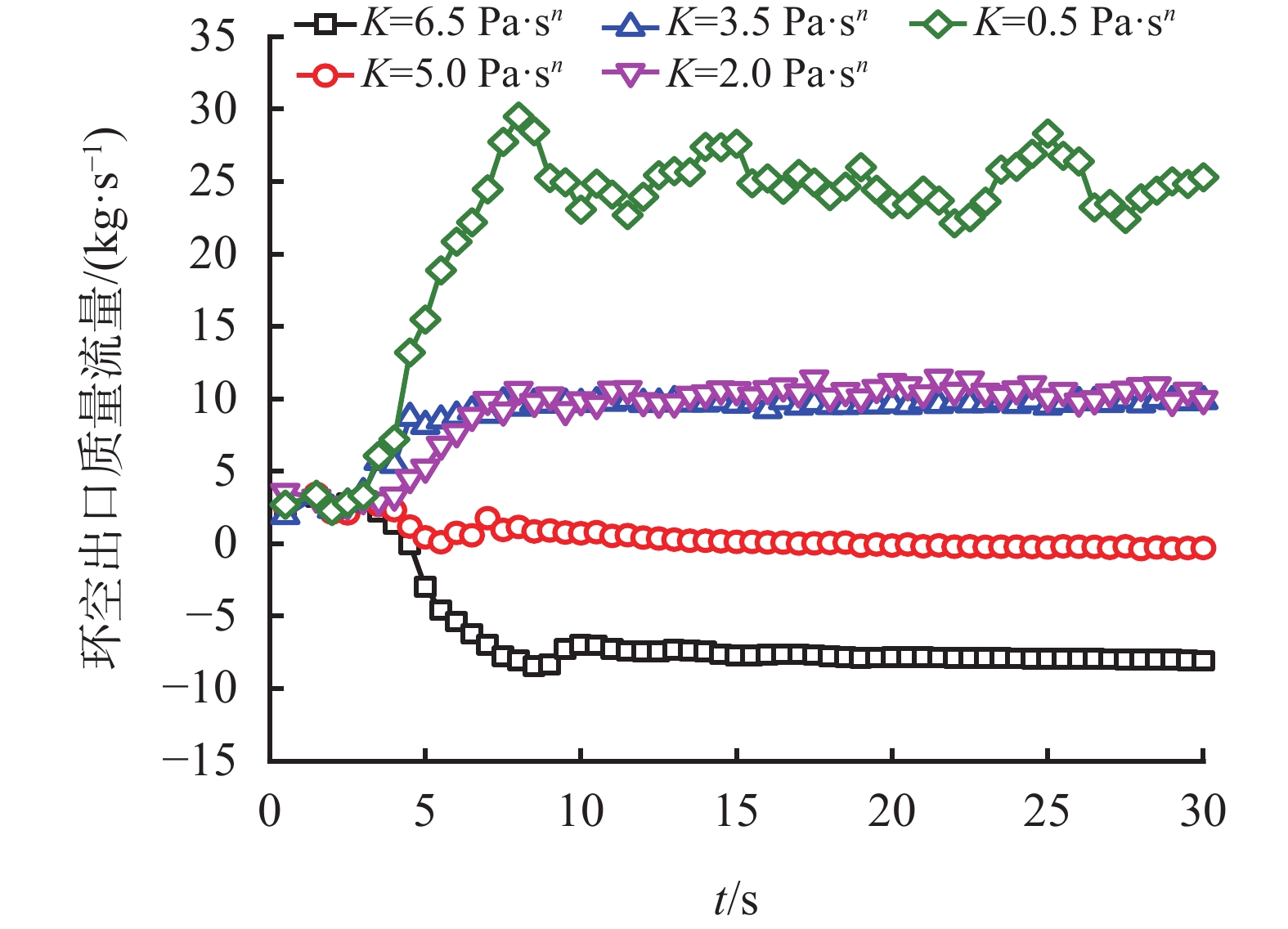
裂缝出口端体积分布和速度分布结果如图6所示,10 s后K为6.5、5、3.5、2 Pa·sn的堵漏浆出口平均流速基本稳定且逐渐上升,分别为10.4、14.3、19.2和24.1 m/s,对应的出口体积分数逐渐下降,分别达到0.985、0.946、0.734和0.547;K为0.5 Pa·sn堵漏浆10 s后裂缝出口流速和体积分数呈现周期波动特性,10~30 s出口平均流速为27.0 m/s,平均体积分数为0.394。
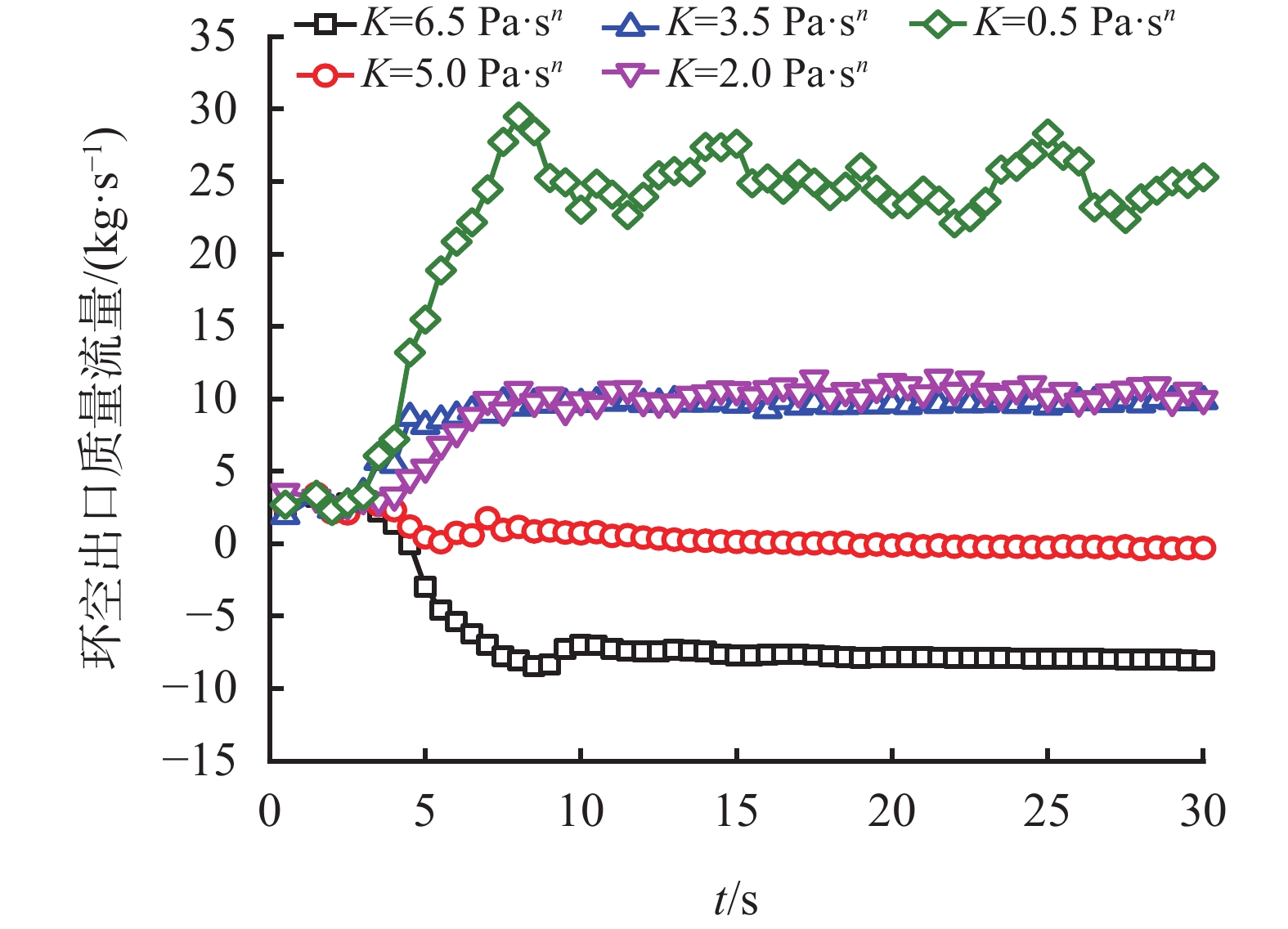
低稠度系数堵漏浆在注入过程中,漏失速度过大,环空钻井液会持续流入钻杆下部井筒。如图7所示,正环空质量流量表示钻井液流入(回流),负环空质量流量表示井筒流体流出。随着稠度系数降低,环空出口质量流量由负转正,其中K为5 Pa·sn的堵漏浆质量流量达到临界点,此时的质量流量约为0。K为3.5、2、和0.5 Pa·sn的堵漏浆注入时,大量环空上部钻井液回流至底部井筒,持续形成共混层,这也解释了图5中低稠度堵漏浆难以形成完整堵漏浆段塞的现象。
2.3 流性指数影响
考察剪切稀释性影响时,动切力τ0和稠度系数K分别固定为5 Pa和6.5 Pa·sn。
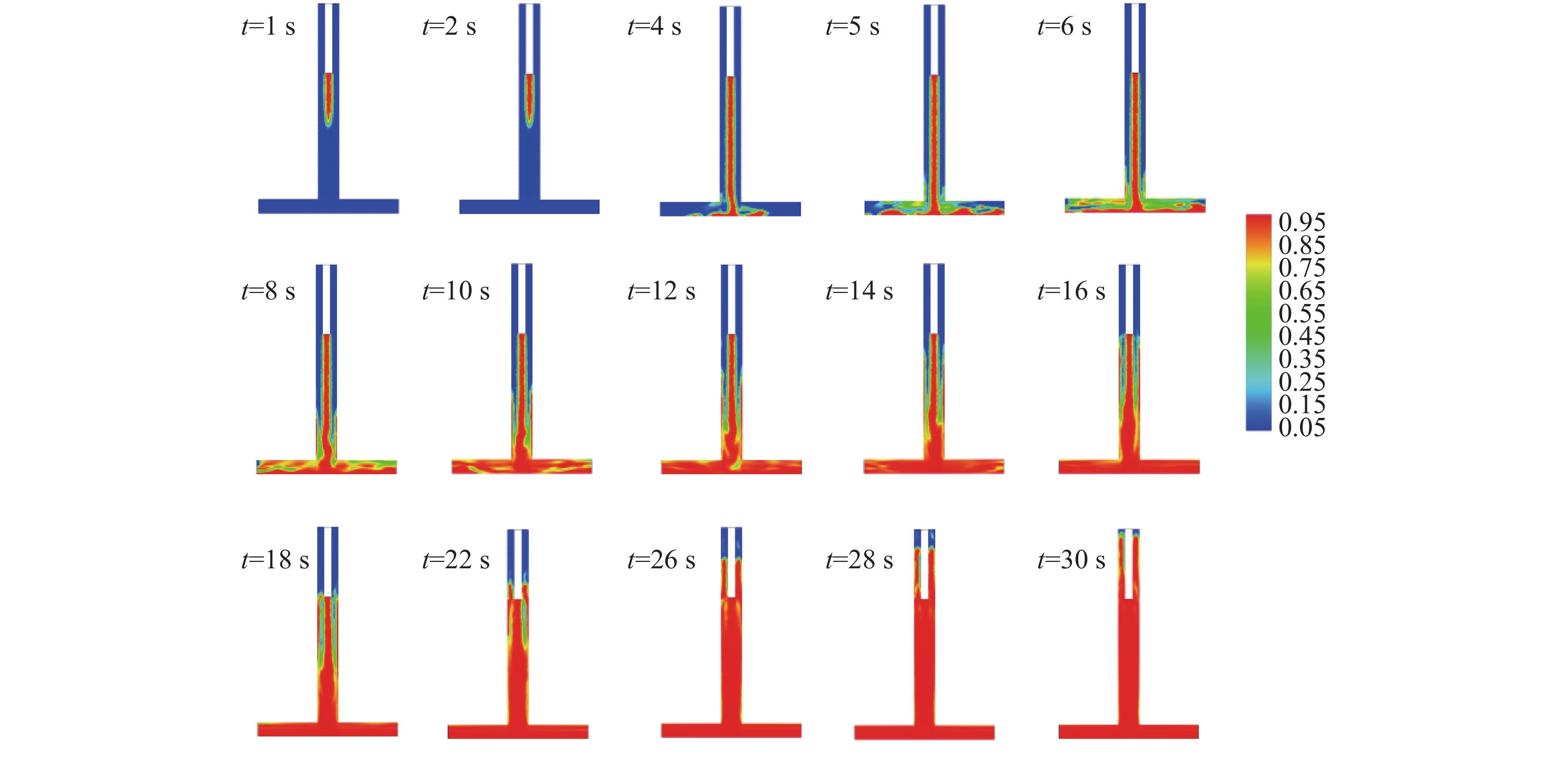
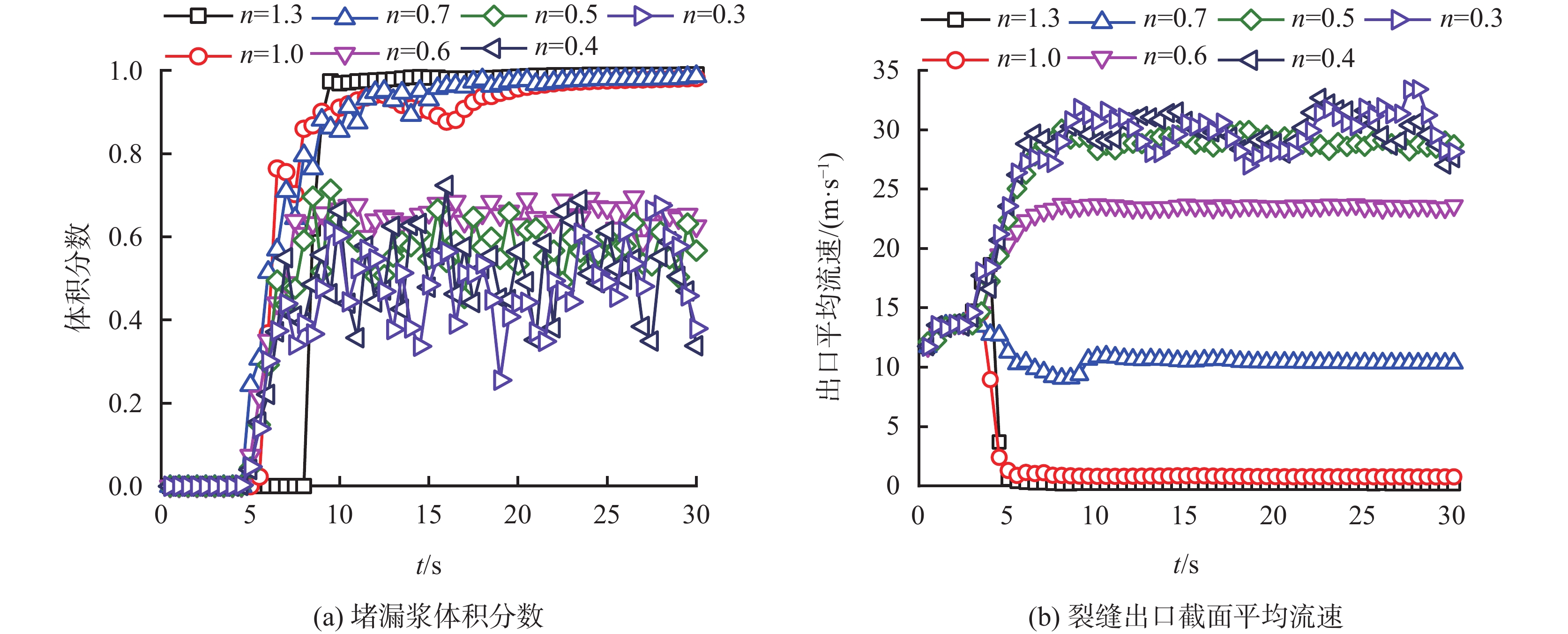
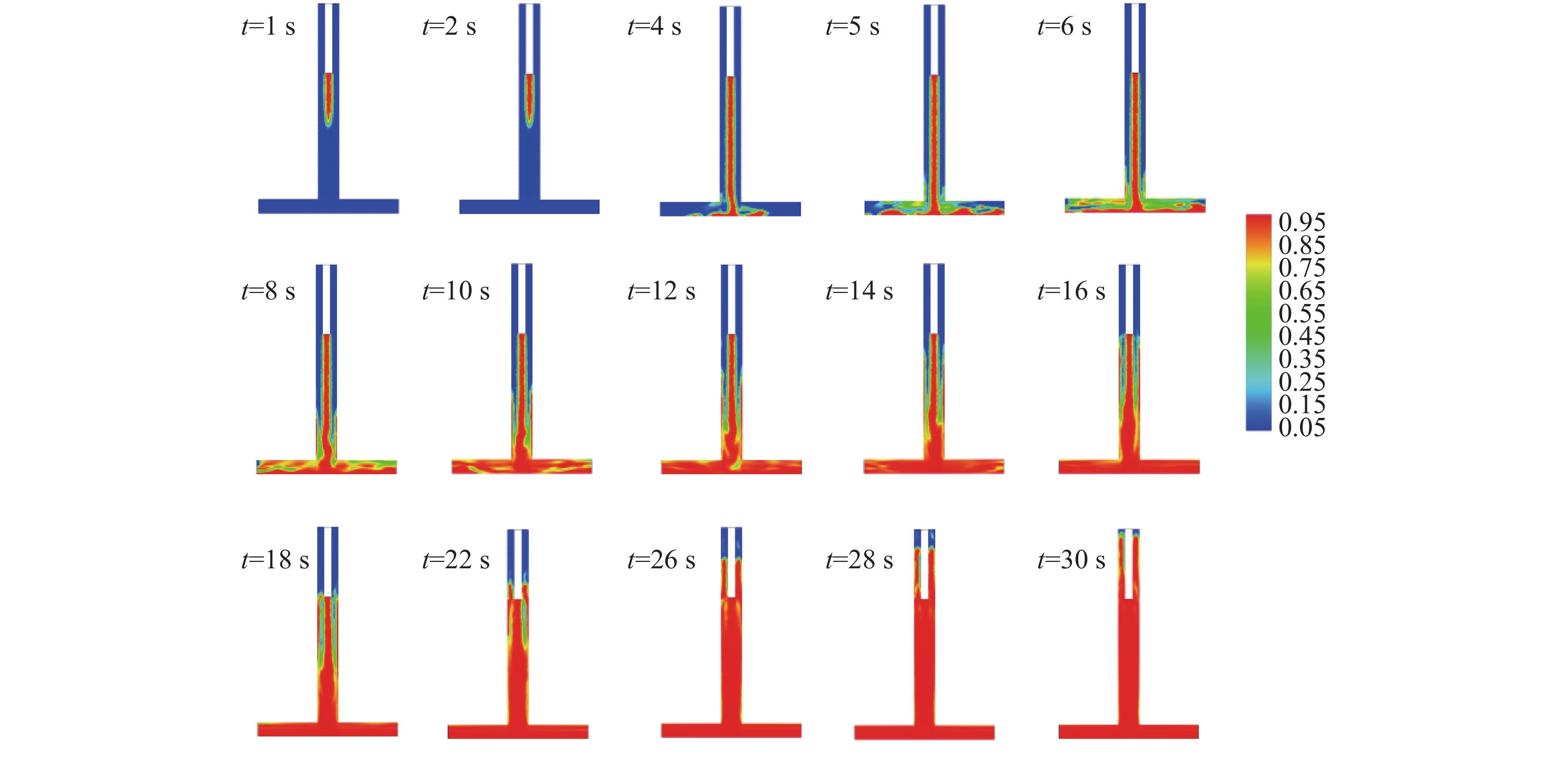
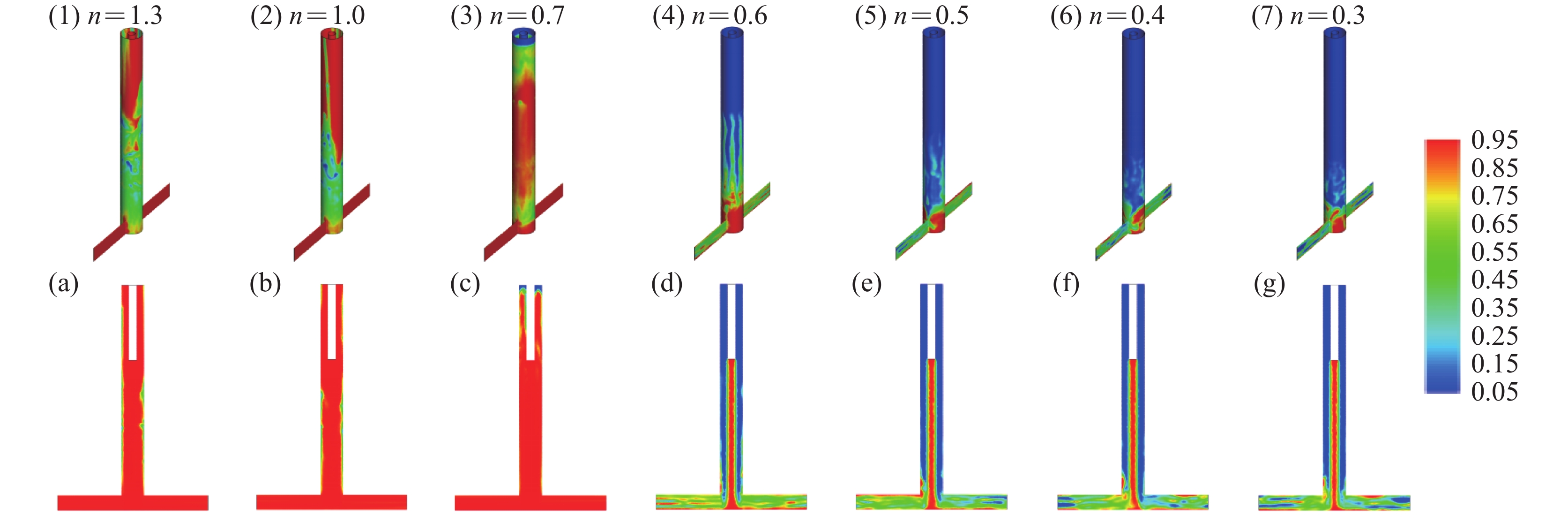
流性指数对堵漏浆驻留性能影响明显,n值越小,裂缝流速越大,驻留能力越差,n≥1时驻留效果提升显著。图8显示了30 s时不同n值堵漏浆在井筒中分布情况,当n≥0.7,堵漏浆可在井筒内形成整段堵漏浆段塞。图9显示了n=1.3堵漏浆的流态随时间变化,该剪切增稠堵漏浆在裂缝和井筒中呈现明显的段塞流,例如t=5 s后,裂缝中堵漏浆平行顶替钻井液,井筒中堵漏浆呈现段塞状,这与n=0.7堵漏浆的流态有明显区别(图4)区别较大。
流性指数n越小,裂缝出口流速越大,堵漏浆体积分数越小。如图10(a),不同n值堵漏浆挤注下,裂缝出口流速在t=10 s左右基本稳定,剪切增稠堵漏浆裂缝出口流速远低于剪切稀释型堵漏浆。例如t=30 s时,n=1.3、1.0、0.7、0.6的堵漏浆裂缝出口流速分别为0.18、0.75、10.3和23.6 m/s,剪切增稠堵漏浆在裂缝中运移速度更低,易在近井壁形成完整固结层,与图10(b)结果相对应。
2.4 动切力影响
K和n分别固定为5 Pa和6.5 Pa·sn,如图11所示,动切力对堵漏浆井下运移状态影响不大。
2.5 稠度系数与流性指数影响结果对比
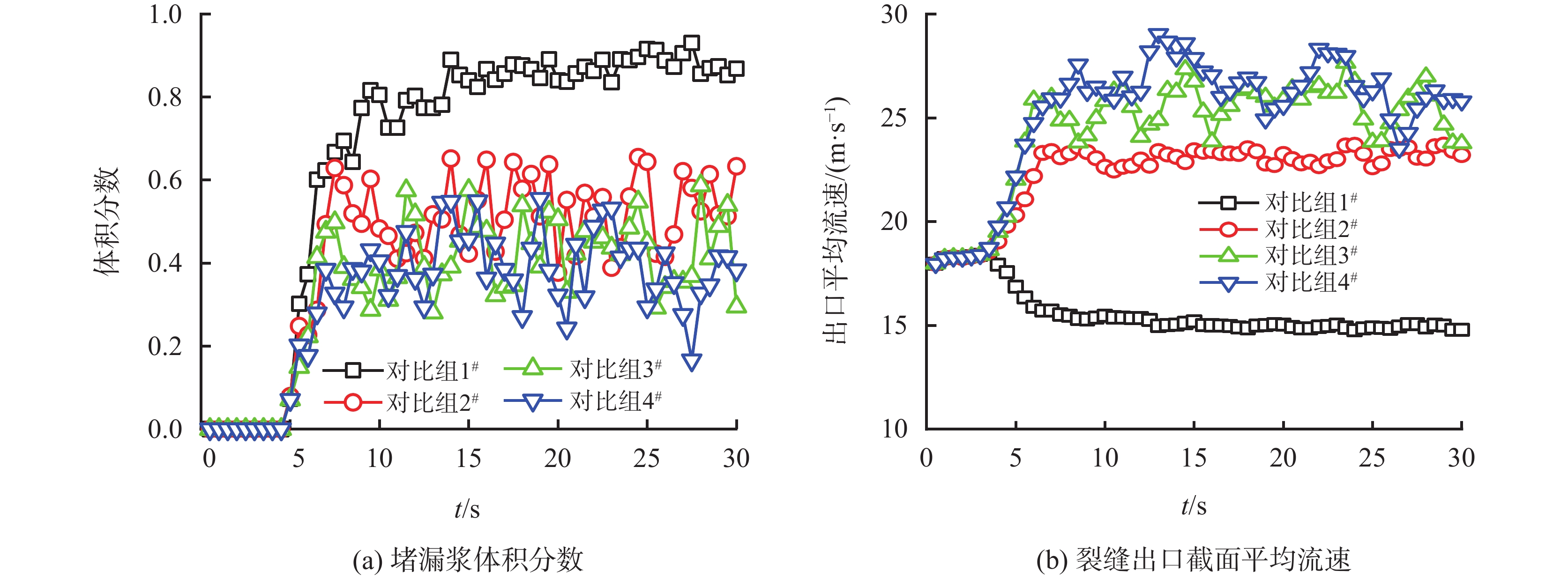
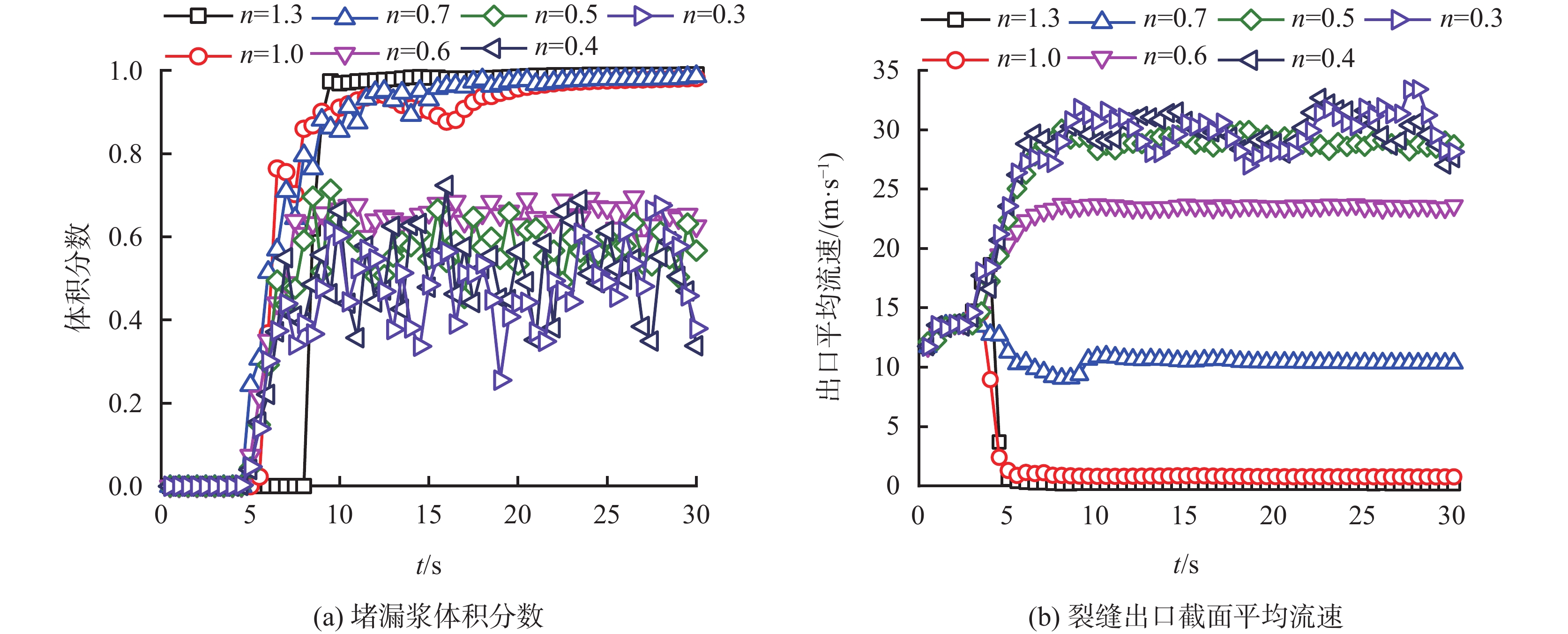
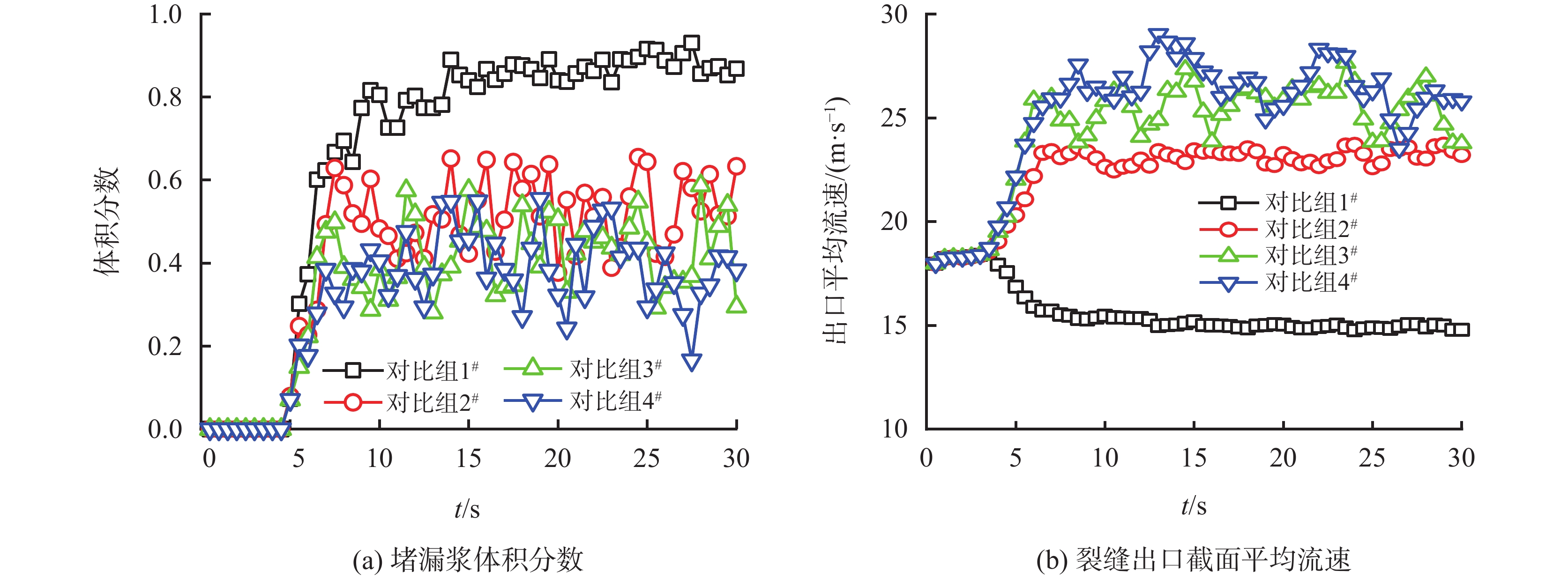
在水泥、树脂的流变调控中,稠度系数K和流性指数n往往同时发生变化。一般而言,稠度系数K越大, 流性指数n越小。为了对比2个流变参数对固化堵漏浆裂缝驻留能力的重要性,设置了4组流变参数(如表2所示),其稠度系数K由0.5 Pa·sn增加至10 Pa·sn,对应的流性指数n由1降低至0.3,仿真模拟结果如图12所示。
表 2 对比组固化堵漏浆流变性能参数对比组 K/Pa·sn n τ0/Pa 1# 0.5 1.0 0.3 2# 2.0 0.7 1.0 3# 5.0 0.5 2.5 4# 10.0 0.3 5.0 如图12所示,流性指数n对固化堵漏浆裂缝驻留能力的影响更明显。如图12(a),裂缝出口堵漏浆体积分数随着流性指数的降低而下降。例如t=30 s时,1#对比组的堵漏浆体积分数在90%以上,能在裂缝中形成完整的段塞;而4#对比组的堵漏浆体积分数仅为40%左右,在裂缝中无法形成完整的段塞;同时,裂缝出口流速随着流性指数的降低而增高,在t=30 s时,1#~4#对比组对应的裂缝出口流速分别为14.7、23.2、23.7和25.8 m/s。
因此为了提升固化堵漏浆在裂缝中驻留能力,针对现场固化堵漏浆流变性能优化时,应该优先考虑提升堵漏浆流性指数,而不是稠度系数。
3. 结论
1. 在水泥、树脂等交联固化堵漏施工中,井筒和裂缝近井壁处能否形成完整固结段塞,是堵漏成功与否的关键。在固化堵漏浆挤注过程中,通过调整堵漏浆性能和工艺优化,在裂缝和井筒底部内形成段塞流,减少混浆段体积,是建立完整固结段塞的前提。通过建立三维井筒-裂缝模型,采用VOF两相流仿真模拟方法,定量研究了堵漏浆密度、流变性参数对固化堵漏浆在注入过程中井下运移规律影响。
2. 堵漏浆密度对其驻留性能影响较小,不同密度堵漏浆在裂缝中体积分布和流速基本一致;堵漏浆稠度系数和剪切稀释性对其裂缝驻留和填充性能影响显著,动切力对运移性能无影响。稠度系数越低或剪切稀释性越强,堵漏浆在裂缝中流速越快,低于临界值后,堵漏浆将无法在井筒和裂缝中形成完整段塞流动,一直以堵漏浆-钻井液混合流动形式存在,牛顿型和剪切增稠型堵漏浆易在裂缝中形成段塞流动,便于形成完整固化层。
3. 根据仿真计算结果,针对大裂缝恶性井漏现场堵漏,固化堵漏浆可设计成高黏度或低剪切稀释性,并优先保证低剪切稀释性,以便井筒和裂缝中形成完整段塞,提高一次堵漏成功率。
-
表 1 三维井筒-裂缝仿真模型
参数 梯度设置 堵漏浆密度/(g·cm−3) 1.1、1.2、1.3、1.4、1.5 堵漏浆流变性能 τ0/Pa 0、1.5、3.0、5.0、6.5 K/Pa·sn 0.5、2.0、3.5、5.0、6.5 n 0.4、0.5、0.6、0.7、1.0、1.3 表 2 对比组固化堵漏浆流变性能参数
对比组 K/Pa·sn n τ0/Pa 1# 0.5 1.0 0.3 2# 2.0 0.7 1.0 3# 5.0 0.5 2.5 4# 10.0 0.3 5.0 -
[1] 孙金声,白英睿,程荣超,等. 裂缝性恶性井漏地层堵漏技术研究进展与展望[J]. 石油勘探与开发,2021,48(3):630-638.SUN Jinsheng, BAI Yingrui, CHENG Rongchao, et al. Research progress and prospect of plugging technologies for fractured formation with severe lost circulation[J]. Petroleum Exploration and Development, 2021, 48(3):630-638. [2] 张志磊,胡百中,卞维坤,等. 昭通页岩气示范区井漏防治技术与实践[J]. 钻井液与完井液,2020,37(1):38-45.ZHANG Zhilei, HU Baizhong, BIAN Weikun, et al. Mud loss control techniques and practices in Zhaotong demonstration zone of shale gas drilling[J]. Drilling Fluid & Completion Fluid, 2020, 37(1):38-45. [3] 谢春来,胡清富,张凤臣,等. 伊拉克哈法亚油田Mishrif组碳酸盐岩储层防漏堵漏技术[J]. 石油钻探技术,2021,49(1):41-46. doi: 10.11911/syztjs.2020125XIE Chunlai, HU Qingfu, ZHANG Fengchen, et al. Antileaking and lost circulation control technology for the Mishrif carbonate reservoir in the Halfaya oilfield of Iraq[J]. Petroleum Drilling Techniques, 2021, 49(1):41-46. doi: 10.11911/syztjs.2020125 [4] 李文博,李公让. 可控化聚合物凝胶堵漏材料的研究进展[J]. 钻井液与完井液,2021,38(2):133-141. doi: 10.3969/j.issn.1001-5620.2021.02.001LI Wenbo, LI Gongrang. Research progress of controllable polymer gel lost circulation materials[J]. Drilling Fluid & Completion Fluid, 2021, 38(2):133-141. doi: 10.3969/j.issn.1001-5620.2021.02.001 [5] 郭锦棠,杜江波,冯杰,等. 高温高压钻井用堵漏材料的制备及性能研究[J]. 天津大学学报(自然科学与工程技术版),2021,54(6):593-598.GUO Jintang, DU Jiangbo, FENG Jie, et al. Preparation and performance of plugging material for high-temperature and high-pressure drilling[J]. Journal of Tianjin University (Science and Technology) , 2021, 54(6):593-598. [6] 张浩,佘继平,杨洋,等. 可酸溶固化堵漏材料的封堵及储层保护性能[J]. 油田化学,2020,37(4):581-586,592.ZHANG Hao, SHE Jiping, YANG Yang, et al. Plugging and formation damage control performance of a lost circulation material[J]. Oilfield chemistry, 2020, 37(4):581-586,592. [7] 赵启阳,邓慧,王伟,等. 一种可固化堵漏工作液的室内研究[J]. 钻井液与完井液,2013,30(1):41-44. doi: 10.3969/j.issn.1001-5620.2013.01.011ZHAO Qiyang, DENG Hui, WANG Wei, et al. Laboratory study on curing lost circulation control fluid[J]. Drilling Fluid & Completion Fluid, 2013, 30(1):41-44. doi: 10.3969/j.issn.1001-5620.2013.01.011 [8] 孙金声,赵震,白英睿,等. 智能自愈合凝胶研究进展及在钻井液领域的应用前景[J]. 石油学报,2020,41(12):1706-1718. doi: 10.7623/syxb202012023SUN Jinsheng, ZHAO Zhen, BAI Yingrui, et al. Progress in research of intelligent self-healing gel and its application prospects in the field of drilling fluid[J]. Acta Petrolei Sinica, 2020, 41(12):1706-1718. doi: 10.7623/syxb202012023 [9] XU B, ZHOU S, HE F, et al. Using particle image velocimetry to evaluate the displacement efficiency of drilling mud in horizontal well cementing[J]. Journal of Petroleum Science and Engineering, 2020, 195(2020):107647-107655. [10] WANG Jingtang, SUN Baojiang, LI Hao, et al. Numerical simulation of cementing displacement interface stability of extended reach wells[J]. Journal of Hydrodynamics, 2018, 30(3):420-432. doi: 10.1007/s42241-018-0051-4 [11] 陶谦,周仕明,张晋凯,等. 水泥浆流变性对水平井固井顶替界面的影响——基于天河一号大规模集群计算平台的数值模拟[J]. 石油钻采工艺,2017,39(2):185-191.TAO Qian, ZHOU Shiming, ZHANG Jinkai, et al. Effect of rheological property of slurry on cementing displacement interface of horizontal well: the numerical simulation based on large-scale cluster computing platform Tianhe-1[J]. Oil Drilling & Production Technology, 2017, 39(2):185-191. [12] 杨谋,唐大千,袁中涛,等. 固井注水泥浆顶替效率评估的新模型[J]. 天然气工业,2019,39(6):115-122. doi: 10.3787/j.issn.1000-0976.2019.06.013YANG Mou, TANG Daqian, YUAN Zhongtao, et al. A new model for evaluating the displacement efficiency of cement slurry[J]. Natural Gas Industry, 2019, 39(6):115-122. doi: 10.3787/j.issn.1000-0976.2019.06.013 [13] 魏凯, 褚冰川, 包莉军, 等. 基于相场法的偏心环空注水泥顶替过程数值模拟 [J]. 钻采工艺, 2020, 43(3): 123-126.WEI Kai, CHU Binchuan, BAO Lijun, et al. Numerical simulation of cementing displacement process in eccentric annulus based on phase field method[J]. Drilling & Production Technology, 2020, 34(3): 123-126. 期刊类型引用(3)
1. 姚海雷,刘飞,王学武,代晓东,张程翔,杨剑,孟嘉孟,刘畅,王阳. 可逆乳状液多次重复可逆转化能力研究. 山东化工. 2022(14): 56-58 .  百度学术
百度学术2. 沈启航,梁大川,王瑜,杨阳. 基于乳化剂EMUL-GP可逆乳化钻井液的研制与性能评价. 化学世界. 2020(04): 298-304 .  百度学术
百度学术3. 刘飞,王彦玲,王学武,代晓东,王硕. 酸碱质量分数对pH控制的可逆乳状液转相的影响. 石油化工高等学校学报. 2020(04): 49-56 .  百度学术
百度学术其他类型引用(2)
-





 下载:
下载:
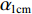
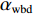

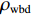
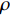
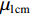
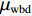


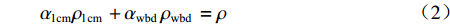
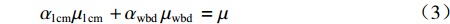

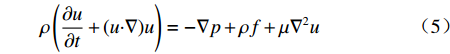












 下载:
下载:
 百度学术
百度学术